
	Advertisement

Geometry Puzzle (update)

Last week I posted a geometry puzzle. The goal was to find the dimensions of the inscribed rectangle that has the same area as an inscribed equilateral triangle. I proposed a solution (you can read my solution here), but was not entirely happy with the complexity of it.

I asked if any of my readers could suggest an alternative way of solving. A couple of you emailed/messaged me suggesting a trigonometric way of calculating the answer (that does not require the solving of a quartic equations.
[Thanks: Andrew Lundeen and Matt Barsalou]

We can define α as the angle between the diameter of the circle (also the hypotenuse of the rectangle), and the base of the rectangle. This gives us two simple formula for the height and width.

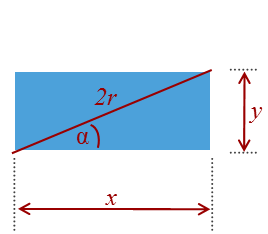Multiplying these two together we get the area of the rectangle.

Dusting off the old-faithful double angle trig identity:

We can simplify to an equation with just one angle:

From before, we know the area of the triangle (see equation 5 from the previous article), and we can equate these and simplify.

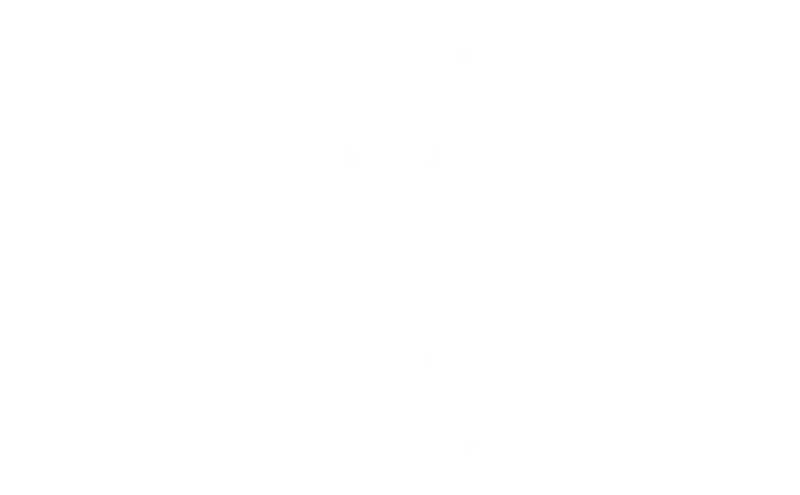
We end up with a simple formula for the angle. Now that we have this angle we can insert it into the formulae for x and y to give the solution derived using a trigonometric approach.

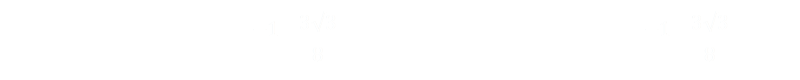
You can find a complete list of all the articles here. Click here to receive email alerts on new articles.