Chicken Nugget Numbers
 |
McDonalds® sell chicken nuggets. They sell nuggets in packs of: six, nine, and twenty.* *I know they now also sell them in packs of four in Happy Meals, and occasionally in packs of ten, but the purpose of this article is to describe math problems, not to learn about the marketing practices of a fast food chain! |
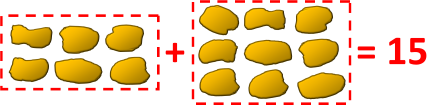
If you are hungry, and decide you want to eat exactly fifteen nuggets, you can buy one pack of six nuggets, and one pack of nine.
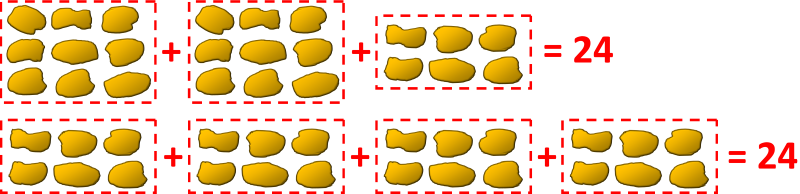
If you are extra hungry, and decide you want to eat twenty-four nuggets you can either purchase two packs of nine and one pack of six, or you could purchase four packs of six.
If you wanted to eat twenty-five nuggets, there’s no way to buy the exact number needed; you’d either have to finish hungry, or buy excess and have some left over.
There are certain numbers of nuggets it’s possible to make, and certain numbers it is impossible to make.
Possible Nugget Numbers
Here is a list of all the different size of nugget meals it is possible to make (using packs of 6,9 and 20), and those that is not possible to make, in an ascending order.
|
|
Something interesting happens. After 43 nuggets, it is possible to fill any order request exactly. The number 43 is the largest number of nuggets it is not possible to make using packs of six, nine and twenty. This special number if given the name of a Frobenius Number and, because others also use the same example I used above, it is commonly called a Chicken Nugget Number.
For a set of integers, there can be a finite set of numbers that cannot be created by summing multiples of members of this set. The largest number it is impossible to make is called the Frobenius Number.
Starting with two
We can start to see why this phenomenon happens if we simplify things to have just two nugget sizes. Imagine that nuggets are only sold in packs of three and packs of eleven.
As we have packs of three, we know that every multiple of three is possible to make (similarly, every multiple of eleven). However, we call also make every third number after eleven, and then every third number after twenty-two (by taking two boxes of eleven, then using as many boxes of three as needed). These combs of attainable numbers soon overlap and we find we can make any number after a certain point.

It was shown in 1884 by the mathematician James Sylvester that for two relatively prime numbers: p, q, then every integer n ≥ (p-1)(q-1) can be formed, and this the largest number that cannot be made is (p-1)(q-1)-1 = pq-p-q
Thus, for boxes of three and eleven nuggets, the Frobenius number is 19.
For sets of numbers greater than two, however, no explicit formula is known. These numbers are named after Ferdinand Georg Frobenius (1849-1917), who first investigated them.
Causing trouble at the Golden Arches?
 Image: Chris Jones Image: Chris Jones |
Now that you know about Frobenius, you can cause trouble* at your local drive-through by asking for "Sixty-one chicken nuggets please!" “Could I order sixty-one chicken nuggets please?” I'm pretty sure the staff will politely inform you that you can't buy sixty-one nuggets, and that they are sold only in boxes of twenty. They will tell you that they can sell you sixty nuggets, but not sixty-one. I'm sure if you insist that you want to buy sixty-one they will insist that they can only sell you sixty. After a couple of rounds of backwards and forwards you can drop the bombshell that, "Actually, you can sell me sixty-one nuggets. All you need to do is sell me two boxes of twenty, two boxes of six, and one box of nine!"
*Actually - Please do not do this. It's not big, and it's not clever! |
|
WTH? As I was researching this article I discovered that, sometimes, McDonalds® sells its McNuggets in boxes of ten. When I first glanced at their packaging, I was convinced there was a mistake. Did they really print the box depicting eleven nuggets on the front of a box advertised as ten? (see right) However, closer examination reveals that the two nuggest in the lower left #5, and #8 are actually just one nugget that has been cut in half.
The McDonalds name, Golden Arches logo, and the product name Chicken McNuggets are all registered trademarks owned by the McDonalds Corporation. |
 |
Coins and Postage Stamps
An analogous problem occurs with the selection of denominations for coins and postage stamps. What values should be selected as the denominations for coins?

If we have to break a dollar, we want to be able to give change for any value of cents needed. Here, however, the problem is slightly different. We know, because we have the penny, which is a $0.01 coin, that we can make any denomination needed. However, it would be nice if we could minimize the number of coins needed (I'm sure you'd be a little annoyed if you received $0.99 in change all in pennies!)
Whilst in the the USA there is a half-dollar coin, it is rarely used in everyday circulation. The coins used regularly are the penny, nickel, dime and quarter ($0.01, $0.05, $0.10 and $0.25).
Below is a table of how these four common coins can be used to make change. For each value of change, I've shown the number of solutions (the distinct number of ways that this value of change can be created), the minimum number of coins that can be used to make this change, and finally, a description of what this minimum solution is.
e.g. There are nine possible ways to make change for $0.21: 21×$0.01 or 1×$0.05, 16×$0.01 or 2×$0.05, 11×$0.01 or 3×$0.05, 6×$0.01 or 4×$0.05, 1×$0.01 or 1×$0.10, 11×$0.01 or 1×$0.10, 1×$0.05, 6×$0.01 or 1×$0.10, 2×$0.05, 1×$0.01 or 2×$0.10, 1×$0.01, of these, the solution that uses the smallest number of coins is 2×$0.10, 1×$0.01, and this uses just three coins.
| Change | # sol | Min | Best Solution |
|---|---|---|---|
| $0.01 | 1 | 1 | 1×$0.01 |
| $0.02 | 1 | 2 | 2×$0.01 |
| $0.03 | 1 | 3 | 3×$0.01 |
| $0.04 | 1 | 4 | 4×$0.01 |
| $0.05 | 2 | 1 | 1×$0.05 |
| $0.06 | 2 | 2 | 1×$0.05, 1×$0.01 |
| $0.07 | 2 | 3 | 1×$0.05, 2×$0.01 |
| $0.08 | 2 | 4 | 1×$0.05, 3×$0.01 |
| $0.09 | 2 | 5 | 1×$0.05, 4×$0.01 |
| $0.10 | 4 | 1 | 1×$0.10 |
| $0.11 | 4 | 2 | 1×$0.10, 1×$0.01 |
| $0.12 | 4 | 3 | 1×$0.10, 2×$0.01 |
| $0.13 | 4 | 4 | 1×$0.10, 3×$0.01 |
| $0.14 | 4 | 5 | 1×$0.10, 4×$0.01 |
| $0.15 | 6 | 2 | 1×$0.10, 1×$0.05 |
| $0.16 | 6 | 3 | 1×$0.10, 1×$0.05, 1×$0.01 |
| $0.17 | 6 | 4 | 1×$0.10, 1×$0.05, 2×$0.01 |
| $0.18 | 6 | 5 | 1×$0.10, 1×$0.05, 3×$0.01 |
| $0.19 | 6 | 6 | 1×$0.10, 1×$0.05, 4×$0.01 |
| $0.20 | 9 | 2 | 2×$0.10 |
| $0.21 | 9 | 3 | 2×$0.10, 1×$0.01 |
| $0.22 | 9 | 4 | 2×$0.10, 2×$0.01 |
| $0.23 | 9 | 5 | 2×$0.10, 3×$0.01 |
| $0.24 | 9 | 6 | 2×$0.10, 4×$0.01 |
| $0.25 | 13 | 1 | 1×$0.25 |
| $0.26 | 13 | 2 | 1×$0.25, 1×$0.01 |
| $0.27 | 13 | 3 | 1×$0.25, 2×$0.01 |
| $0.28 | 13 | 4 | 1×$0.25, 3×$0.01 |
| $0.29 | 13 | 5 | 1×$0.25, 4×$0.01 |
| $0.30 | 18 | 2 | 1×$0.25, 1×$0.05 |
| $0.31 | 18 | 3 | 1×$0.25, 1×$0.05, 1×$0.01 |
| $0.32 | 18 | 4 | 1×$0.25, 1×$0.05, 2×$0.01 |
| $0.33 | 18 | 5 | 1×$0.25, 1×$0.05, 3×$0.01 |
| $0.34 | 18 | 6 | 1×$0.25, 1×$0.05, 4×$0.01 |
| $0.35 | 24 | 2 | 1×$0.25, 1×$0.10 |
| $0.36 | 24 | 3 | 1×$0.25, 1×$0.10, 1×$0.01 |
| $0.37 | 24 | 4 | 1×$0.25, 1×$0.10, 2×$0.01 |
| $0.38 | 24 | 5 | 1×$0.25, 1×$0.10, 3×$0.01 |
| $0.39 | 24 | 6 | 1×$0.25, 1×$0.10, 4×$0.01 |
| $0.40 | 31 | 3 | 1×$0.25, 1×$0.10, 1×$0.05 |
| $0.41 | 31 | 4 | 1×$0.25, 1×$0.10, 1×$0.05, 1×$0.01 |
| $0.42 | 31 | 5 | 1×$0.25, 1×$0.10, 1×$0.05, 2×$0.01 |
| $0.43 | 31 | 6 | 1×$0.25, 1×$0.10, 1×$0.05, 3×$0.01 |
| $0.44 | 31 | 7 | 1×$0.25, 1×$0.10, 1×$0.05, 4×$0.01 |
| $0.45 | 39 | 3 | 1×$0.25, 2×$0.10 |
| $0.46 | 39 | 4 | 1×$0.25, 2×$0.10, 1×$0.01 |
| $0.47 | 39 | 5 | 1×$0.25, 2×$0.10, 2×$0.01 |
| $0.48 | 39 | 6 | 1×$0.25, 2×$0.10, 3×$0.01 |
| $0.49 | 39 | 7 | 1×$0.25, 2×$0.10, 4×$0.01 |
| $0.50 | 49 | 2 | 2×$0.25 |
| $0.51 | 49 | 3 | 2×$0.25, 1×$0.01 |
| $0.52 | 49 | 4 | 2×$0.25, 2×$0.01 |
| $0.53 | 49 | 5 | 2×$0.25, 3×$0.01 |
| $0.54 | 49 | 6 | 2×$0.25, 4×$0.01 |
| $0.55 | 60 | 3 | 2×$0.25, 1×$0.05 |
| $0.56 | 60 | 4 | 2×$0.25, 1×$0.05, 1×$0.01 |
| $0.57 | 60 | 5 | 2×$0.25, 1×$0.05, 2×$0.01 |
| $0.58 | 60 | 6 | 2×$0.25, 1×$0.05, 3×$0.01 |
| $0.59 | 60 | 7 | 2×$0.25, 1×$0.05, 4×$0.01 |
| $0.60 | 73 | 3 | 2×$0.25, 1×$0.10 |
| $0.61 | 73 | 4 | 2×$0.25, 1×$0.10, 1×$0.01 |
| $0.62 | 73 | 5 | 2×$0.25, 1×$0.10, 2×$0.01 |
| $0.63 | 73 | 6 | 2×$0.25, 1×$0.10, 3×$0.01 |
| $0.64 | 73 | 7 | 2×$0.25, 1×$0.10, 4×$0.01 |
| $0.65 | 87 | 4 | 2×$0.25, 1×$0.10, 1×$0.05 |
| $0.66 | 87 | 5 | 2×$0.25, 1×$0.10, 1×$0.05, 1×$0.01 |
| $0.67 | 87 | 6 | 2×$0.25, 1×$0.10, 1×$0.05, 2×$0.01 |
| $0.68 | 87 | 7 | 2×$0.25, 1×$0.10, 1×$0.05, 3×$0.01 |
| $0.69 | 87 | 8 | 2×$0.25, 1×$0.10, 1×$0.05, 4×$0.01 |
| $0.70 | 103 | 4 | 2×$0.25, 2×$0.10 |
| $0.71 | 103 | 5 | 2×$0.25, 2×$0.10, 1×$0.01 |
| $0.72 | 103 | 6 | 2×$0.25, 2×$0.10, 2×$0.01 |
| $0.73 | 103 | 7 | 2×$0.25, 2×$0.10, 3×$0.01 |
| $0.74 | 103 | 8 | 2×$0.25, 2×$0.10, 4×$0.01 |
| $0.75 | 121 | 3 | 3×$0.25 |
| $0.76 | 121 | 4 | 3×$0.25, 1×$0.01 |
| $0.77 | 121 | 5 | 3×$0.25, 2×$0.01 |
| $0.78 | 121 | 6 | 3×$0.25, 3×$0.01 |
| $0.79 | 121 | 7 | 3×$0.25, 4×$0.01 |
| $0.80 | 141 | 4 | 3×$0.25, 1×$0.05 |
| $0.81 | 141 | 5 | 3×$0.25, 1×$0.05, 1×$0.01 |
| $0.82 | 141 | 6 | 3×$0.25, 1×$0.05, 2×$0.01 |
| $0.83 | 141 | 7 | 3×$0.25, 1×$0.05, 3×$0.01 |
| $0.84 | 141 | 8 | 3×$0.25, 1×$0.05, 4×$0.01 |
| $0.85 | 163 | 4 | 3×$0.25, 1×$0.10 |
| $0.86 | 163 | 5 | 3×$0.25, 1×$0.10, 1×$0.01 |
| $0.87 | 163 | 6 | 3×$0.25, 1×$0.10, 2×$0.01 |
| $0.88 | 163 | 7 | 3×$0.25, 1×$0.10, 3×$0.01 |
| $0.89 | 163 | 8 | 3×$0.25, 1×$0.10, 4×$0.01 |
| $0.90 | 187 | 5 | 3×$0.25, 1×$0.10, 1×$0.05 |
| $0.91 | 187 | 6 | 3×$0.25, 1×$0.10, 1×$0.05, 1×$0.01 |
| $0.92 | 187 | 7 | 3×$0.25, 1×$0.10, 1×$0.05, 2×$0.01 |
| $0.93 | 187 | 8 | 3×$0.25, 1×$0.10, 1×$0.05, 3×$0.01 |
| $0.94 | 187 | 9 | 3×$0.25, 1×$0.10, 1×$0.05, 4×$0.01 |
| $0.95 | 213 | 5 | 3×$0.25, 2×$0.10 |
| $0.96 | 213 | 6 | 3×$0.25, 2×$0.10, 1×$0.01 |
| $0.97 | 213 | 7 | 3×$0.25, 2×$0.10, 2×$0.01 |
| $0.98 | 213 | 8 | 3×$0.25, 2×$0.10, 3×$0.01 |
| $0.99 | 213 | 9 | 3×$0.25, 2×$0.10, 4×$0.01 |
Looking at the above table, the worst case for change takes nine coins. Making change for both $0.94 and $0.99 takes nine coins (and this is if we use the optimal number of coins to make change).
Adding back in the half-dollar
If we add back in the half-dollar, how does this change things? Let's take a look:
| Change | # sol | Min | Best Solution |
|---|---|---|---|
| $0.01 | 1 | 1 | 1×$0.01 |
| $0.02 | 1 | 2 | 2×$0.01 |
| $0.03 | 1 | 3 | 3×$0.01 |
| $0.04 | 1 | 4 | 4×$0.01 |
| $0.05 | 2 | 1 | 1×$0.05 |
| $0.06 | 2 | 2 | 1×$0.05, 1×$0.01 |
| $0.07 | 2 | 3 | 1×$0.05, 2×$0.01 |
| $0.08 | 2 | 4 | 1×$0.05, 3×$0.01 |
| $0.09 | 2 | 5 | 1×$0.05, 4×$0.01 |
| $0.10 | 4 | 1 | 1×$0.10 |
| $0.11 | 4 | 2 | 1×$0.10, 1×$0.01 |
| $0.12 | 4 | 3 | 1×$0.10, 2×$0.01 |
| $0.13 | 4 | 4 | 1×$0.10, 3×$0.01 |
| $0.14 | 4 | 5 | 1×$0.10, 4×$0.01 |
| $0.15 | 6 | 2 | 1×$0.10, 1×$0.05 |
| $0.16 | 6 | 3 | 1×$0.10, 1×$0.05, 1×$0.01 |
| $0.17 | 6 | 4 | 1×$0.10, 1×$0.05, 2×$0.01 |
| $0.18 | 6 | 5 | 1×$0.10, 1×$0.05, 3×$0.01 |
| $0.19 | 6 | 6 | 1×$0.10, 1×$0.05, 4×$0.01 |
| $0.20 | 9 | 2 | 2×$0.10 |
| $0.21 | 9 | 3 | 2×$0.10, 1×$0.01 |
| $0.22 | 9 | 4 | 2×$0.10, 2×$0.01 |
| $0.23 | 9 | 5 | 2×$0.10, 3×$0.01 |
| $0.24 | 9 | 6 | 2×$0.10, 4×$0.01 |
| $0.25 | 13 | 1 | 1×$0.25 |
| $0.26 | 13 | 2 | 1×$0.25, 1×$0.01 |
| $0.27 | 13 | 3 | 1×$0.25, 2×$0.01 |
| $0.28 | 13 | 4 | 1×$0.25, 3×$0.01 |
| $0.29 | 13 | 5 | 1×$0.25, 4×$0.01 |
| $0.30 | 18 | 2 | 1×$0.25, 1×$0.05 |
| $0.31 | 18 | 3 | 1×$0.25, 1×$0.05, 1×$0.01 |
| $0.32 | 18 | 4 | 1×$0.25, 1×$0.05, 2×$0.01 |
| $0.33 | 18 | 5 | 1×$0.25, 1×$0.05, 3×$0.01 |
| $0.34 | 18 | 6 | 1×$0.25, 1×$0.05, 4×$0.01 |
| $0.35 | 24 | 2 | 1×$0.25, 1×$0.10 |
| $0.36 | 24 | 3 | 1×$0.25, 1×$0.10, 1×$0.01 |
| $0.37 | 24 | 4 | 1×$0.25, 1×$0.10, 2×$0.01 |
| $0.38 | 24 | 5 | 1×$0.25, 1×$0.10, 3×$0.01 |
| $0.39 | 24 | 6 | 1×$0.25, 1×$0.10, 4×$0.01 |
| $0.40 | 31 | 3 | 1×$0.25, 1×$0.10, 1×$0.05 |
| $0.41 | 31 | 4 | 1×$0.25, 1×$0.10, 1×$0.05, 1×$0.01 |
| $0.42 | 31 | 5 | 1×$0.25, 1×$0.10, 1×$0.05, 2×$0.01 |
| $0.43 | 31 | 6 | 1×$0.25, 1×$0.10, 1×$0.05, 3×$0.01 |
| $0.44 | 31 | 7 | 1×$0.25, 1×$0.10, 1×$0.05, 4×$0.01 |
| $0.45 | 39 | 3 | 1×$0.25, 2×$0.10 |
| $0.46 | 39 | 4 | 1×$0.25, 2×$0.10, 1×$0.01 |
| $0.47 | 39 | 5 | 1×$0.25, 2×$0.10, 2×$0.01 |
| $0.48 | 39 | 6 | 1×$0.25, 2×$0.10, 3×$0.01 |
| $0.49 | 39 | 7 | 1×$0.25, 2×$0.10, 4×$0.01 |
| $0.50 | 50 | 1 | 1×$0.50 |
| $0.51 | 50 | 2 | 1×$0.50, 1×$0.01 |
| $0.52 | 50 | 3 | 1×$0.50, 2×$0.01 |
| $0.53 | 50 | 4 | 1×$0.50, 3×$0.01 |
| $0.54 | 50 | 5 | 1×$0.50, 4×$0.01 |
| $0.55 | 62 | 2 | 1×$0.50, 1×$0.05 |
| $0.56 | 62 | 3 | 1×$0.50, 1×$0.05, 1×$0.01 |
| $0.57 | 62 | 4 | 1×$0.50, 1×$0.05, 2×$0.01 |
| $0.58 | 62 | 5 | 1×$0.50, 1×$0.05, 3×$0.01 |
| $0.59 | 62 | 6 | 1×$0.50, 1×$0.05, 4×$0.01 |
| $0.60 | 77 | 2 | 1×$0.50, 1×$0.10 |
| $0.61 | 77 | 3 | 1×$0.50, 1×$0.10, 1×$0.01 |
| $0.62 | 77 | 4 | 1×$0.50, 1×$0.10, 2×$0.01 |
| $0.63 | 77 | 5 | 1×$0.50, 1×$0.10, 3×$0.01 |
| $0.64 | 77 | 6 | 1×$0.50, 1×$0.10, 4×$0.01 |
| $0.65 | 93 | 3 | 1×$0.50, 1×$0.10, 1×$0.05 |
| $0.66 | 93 | 4 | 1×$0.50, 1×$0.10, 1×$0.05, 1×$0.01 |
| $0.67 | 93 | 5 | 1×$0.50, 1×$0.10, 1×$0.05, 2×$0.01 |
| $0.68 | 93 | 6 | 1×$0.50, 1×$0.10, 1×$0.05, 3×$0.01 |
| $0.69 | 93 | 7 | 1×$0.50, 1×$0.10, 1×$0.05, 4×$0.01 |
| $0.70 | 112 | 3 | 1×$0.50, 2×$0.10 |
| $0.71 | 112 | 4 | 1×$0.50, 2×$0.10, 1×$0.01 |
| $0.72 | 112 | 5 | 1×$0.50, 2×$0.10, 2×$0.01 |
| $0.73 | 112 | 6 | 1×$0.50, 2×$0.10, 3×$0.01 |
| $0.74 | 112 | 7 | 1×$0.50, 2×$0.10, 4×$0.01 |
| $0.75 | 134 | 2 | 1×$0.50, 1×$0.25 |
| $0.76 | 134 | 3 | 1×$0.50, 1×$0.25, 1×$0.01 |
| $0.77 | 134 | 4 | 1×$0.50, 1×$0.25, 2×$0.01 |
| $0.78 | 134 | 5 | 1×$0.50, 1×$0.25, 3×$0.01 |
| $0.79 | 134 | 6 | 1×$0.50, 1×$0.25, 4×$0.01 |
| $0.80 | 159 | 3 | 1×$0.50, 1×$0.25, 1×$0.05 |
| $0.81 | 159 | 4 | 1×$0.50, 1×$0.25, 1×$0.05, 1×$0.01 |
| $0.82 | 159 | 5 | 1×$0.50, 1×$0.25, 1×$0.05, 2×$0.01 |
| $0.83 | 159 | 6 | 1×$0.50, 1×$0.25, 1×$0.05, 3×$0.01 |
| $0.84 | 159 | 7 | 1×$0.50, 1×$0.25, 1×$0.05, 4×$0.01 |
| $0.85 | 187 | 3 | 1×$0.50, 1×$0.25, 1×$0.10 |
| $0.86 | 187 | 4 | 1×$0.50, 1×$0.25, 1×$0.10, 1×$0.01 |
| $0.87 | 187 | 5 | 1×$0.50, 1×$0.25, 1×$0.10, 2×$0.01 |
| $0.88 | 187 | 6 | 1×$0.50, 1×$0.25, 1×$0.10, 3×$0.01 |
| $0.89 | 187 | 7 | 1×$0.50, 1×$0.25, 1×$0.10, 4×$0.01 |
| $0.90 | 218 | 4 | 1×$0.50, 1×$0.25, 1×$0.10, 1×$0.05 |
| $0.91 | 218 | 5 | 1×$0.50, 1×$0.25, 1×$0.10, 1×$0.05, 1×$0.01 |
| $0.92 | 218 | 6 | 1×$0.50, 1×$0.25, 1×$0.10, 1×$0.05, 2×$0.01 |
| $0.93 | 218 | 7 | 1×$0.50, 1×$0.25, 1×$0.10, 1×$0.05, 3×$0.01 |
| $0.94 | 218 | 8 | 1×$0.50, 1×$0.25, 1×$0.10, 1×$0.05, 4×$0.01 |
| $0.95 | 252 | 4 | 1×$0.50, 1×$0.25, 2×$0.10 |
| $0.96 | 252 | 5 | 1×$0.50, 1×$0.25, 2×$0.10, 1×$0.01 |
| $0.97 | 252 | 6 | 1×$0.50, 1×$0.25, 2×$0.10, 2×$0.01 |
| $0.98 | 252 | 7 | 1×$0.50, 1×$0.25, 2×$0.10, 3×$0.01 |
| $0.99 | 252 | 8 | 1×$0.50, 1×$0.25, 2×$0.10, 4×$0.01 |
With the addition of the half-dollar, we can make optimal change for $0.94 and $.99 in one fewer coins.
In fact this makes total sense (see what I did there?) - The addition of a $0.50 coin can only affect answers for change values greater than $0.49, and for each solution higher than this the optimal number of coins required can be reduced by one (instead of two quarters, a single half-dollar can be used).
Can we do better? Yes, we can. If, instead of a half-dollar, we had a different denomination coin, what coin should that be?
Get rid of the half-dollar and replace it with …
For this exercise, I've kept the four common coins: $0.01, $0.05, $0.10 and $0.25 and experimented with how optimal change would be adjusted if we minted a coin of value ¢N
Below is a table of the results. The column on the left (N) shows the value of the coin that could be minted instead of the $0.50. The 'Worst' column describes the highest number of coins needed when giving (optimal) change. The 'Worst Values' describes which change value(s) this bastard worst count corresponds to. Finally the 'Average Change size' is the average number of coins needed to give change over the entire range of possible change values.
| N | Worst | Worst Values | Avg Change Size |
|---|---|---|---|
| $0.01 | 9 | $0.94, $0.99 | 4.747 |
| $0.02 | 7 | $0.93, $0.94, $0.98, $0.99 | 3.939 |
| $0.03 | 7 | $0.92, $0.94, $0.97, $0.99 | 3.939 |
| $0.04 | 7 | $0.92, $0.97, $0.98 | 3.899 |
| $0.05 | 9 | $0.94, $0.99 | 4.747 |
| $0.06 | 7 | $0.79, $0.84, $0.89, $0.94, $0.98, $0.99 | 4.061 |
| $0.07 | 7 | $0.98 | 3.788 |
| $0.08 | 7 | $0.97 | 3.758 |
| $0.09 | 7 | $0.92, $0.97 | 3.828 |
| $0.10 | 9 | $0.94, $0.99 | 4.747 |
| $0.11 | 7 | $0.89, $0.99 | 3.879 |
| $0.12 | 6 | $0.78, $0.83, $0.89, $0.91, $0.93, $0.94, $0.96, $0.98 | 3.697 |
| $0.13 | 7 | $0.92, $0.97 | 3.838 |
| $0.14 | 6 | $0.82, $0.87, $0.91, $0.93, $0.96, $0.97, $0.98 | 3.717 |
| $0.15 | 9 | $0.99 | 4.545 |
| $0.16 | 6 | $0.79, $0.84, $0.88, $0.93, $0.94, $0.97, $0.99 | 3.768 |
| $0.17 | 6 | $0.83, $0.88, $0.91, $0.96, $0.98, $0.99 | 3.636 |
| $0.18 | 6 | $0.92, $0.99 | 3.545 |
| $0.19 | 6 | $0.91, $0.96, $0.97 | 3.657 |
| $0.20 | 8 | $0.84, $0.89, $0.94, $0.99 | 4.394 |
| $0.21 | 6 | $0.19, $0.39, $0.59, $0.79, $0.99 | 3.707 |
| $0.22 | 6 | $0.19 | 3.525 |
| $0.23 | 6 | $0.19, $0.87 | 3.535 |
| $0.24 | 6 | $0.19, $0.43, $0.67, $0.91 | 3.657 |
| $0.25 | 9 | $0.94, $0.99 | 4.747 |
| $0.26 | 6 | $0.19, $0.24, $0.44, $0.49, $0.69, $0.74, $0.94, $0.99 | 3.737 |
| $0.27 | 6 | $0.19, $0.24, $0.93, $0.98 | 3.576 |
| $0.28 | 6 | $0.19, $0.24, $0.97 | 3.535 |
| $0.29 | 6 | $0.19, $0.24, $0.43, $0.48, $0.67, $0.72, $0.82, $0.91, $0.96 | 3.747 |
| $0.30 | 8 | $0.99 | 4.192 |
| $0.31 | 6 | $0.19, $0.24, $0.49, $0.54, $0.79, $0.84 | 3.626 |
| $0.32 | 6 | $0.19, $0.24 | 3.495 |
| $0.33 | 6 | $0.19, $0.24 | 3.525 |
| $0.34 | 7 | $0.92, $0.97 | 3.869 |
| $0.35 | 8 | $0.94 | 4.141 |
| $0.36 | 6 | $0.19, $0.24, $0.34, $0.54, $0.59, $0.69, $0.89, $0.94 | 3.667 |
| $0.37 | 6 | $0.19, $0.24, $0.34, $0.91, $0.96 | 3.596 |
| $0.38 | 6 | $0.19, $0.24, $0.34, $0.67, $0.72 | 3.616 |
| $0.39 | 7 | $0.73, $0.97 | 3.828 |
| $0.40 | 8 | $0.99 | 4.141 |
| $0.41 | 6 | $0.19, $0.24, $0.34, $0.39, $0.59, $0.64, $0.74, $0.79, $0.99 | 3.687 |
| $0.42 | 6 | $0.19, $0.24, $0.34, $0.39 | 3.576 |
| $0.43 | 6 | $0.19, $0.24, $0.34, $0.39, $0.67, $0.72, $0.82 | 3.657 |
| $0.44 | 7 | $0.68, $0.83 | 3.869 |
| $0.45 | 8 | $0.89 | 4.141 |
| $0.46 | 7 | $0.44, $0.89 | 3.778 |
| $0.47 | 7 | $0.44 | 3.677 |
| $0.48 | 7 | $0.44, $0.92 | 3.798 |
| $0.49 | 8 | $0.93 | 4.141 |
| $0.50 | 8 | $0.94, $0.99 | 4.242 |
| $0.51 | 7 | $0.44, $0.49, $0.94, $0.99 | 3.939 |
| $0.52 | 7 | $0.44, $0.49 | 3.838 |
| $0.53 | 7 | $0.44, $0.49, $0.92, $0.97 | 3.939 |
| $0.54 | 8 | $0.98 | 4.081 |
| $0.55 | 8 | $0.99 | 4.141 |
| $0.56 | 7 | $0.44, $0.49, $0.99 | 3.899 |
| $0.57 | 7 | $0.44, $0.49 | 3.838 |
| $0.58 | 7 | $0.44, $0.49, $0.92, $0.97 | 3.960 |
| $0.59 | 7 | $0.44, $0.49, $0.83, $0.93, $0.98 | 4.020 |
| $0.60 | 7 | $0.44, $0.49, $0.59, $0.79, $0.84, $0.94, $0.99 | 4.091 |
| $0.61 | 7 | $0.44, $0.49, $0.59 | 3.899 |
| $0.62 | 7 | $0.44, $0.49, $0.59 | 3.869 |
| $0.63 | 7 | $0.44, $0.49, $0.59, $0.97 | 3.939 |
| $0.64 | 7 | $0.44, $0.49, $0.59, $0.83, $0.88, $0.98 | 4.051 |
| $0.65 | 7 | $0.44, $0.49, $0.59, $0.64, $0.84, $0.89, $0.99 | 4.091 |
| $0.66 | 7 | $0.44, $0.49, $0.59, $0.64 | 3.939 |
| $0.67 | 7 | $0.44, $0.49, $0.59, $0.64 | 3.929 |
| $0.68 | 7 | $0.44, $0.49, $0.59, $0.64, $0.92 | 4.000 |
| $0.69 | 7 | $0.44, $0.49, $0.59, $0.64, $0.68, $0.88, $0.93 | 4.091 |
| $0.70 | 8 | $0.69 | 4.141 |
| $0.71 | 8 | $0.69 | 4.020 |
| $0.72 | 8 | $0.69 | 4.020 |
| $0.73 | 8 | $0.69 | 4.141 |
| $0.74 | 8 | $0.69 | 4.182 |
| $0.75 | 8 | $0.69, $0.74 | 4.242 |
| $0.76 | 8 | $0.69, $0.74 | 4.141 |
| $0.77 | 8 | $0.69, $0.74 | 4.141 |
| $0.78 | 8 | $0.69, $0.74 | 4.182 |
| $0.79 | 8 | $0.69, $0.74 | 4.202 |
| $0.80 | 8 | $0.69, $0.74 | 4.242 |
| $0.81 | 8 | $0.69, $0.74 | 4.182 |
| $0.82 | 8 | $0.69, $0.74 | 4.202 |
| $0.83 | 8 | $0.69, $0.74 | 4.212 |
| $0.84 | 8 | $0.69, $0.74 | 4.242 |
| $0.85 | 8 | $0.69, $0.74, $0.84 | 4.293 |
| $0.86 | 8 | $0.69, $0.74, $0.84 | 4.263 |
| $0.87 | 8 | $0.69, $0.74, $0.84 | 4.273 |
| $0.88 | 8 | $0.69, $0.74, $0.84 | 4.293 |
| $0.89 | 8 | $0.69, $0.74, $0.84 | 4.333 |
| $0.90 | 8 | $0.69, $0.74, $0.84, $0.89 | 4.394 |
| $0.91 | 8 | $0.69, $0.74, $0.84, $0.89 | 4.384 |
| $0.92 | 8 | $0.69, $0.74, $0.84, $0.89 | 4.394 |
| $0.93 | 8 | $0.69, $0.74, $0.84, $0.89 | 4.424 |
| $0.94 | 8 | $0.69, $0.74, $0.84, $0.89, $0.93 | 4.475 |
| $0.95 | 9 | $0.94 | 4.545 |
| $0.96 | 9 | $0.94 | 4.545 |
| $0.97 | 9 | $0.94 | 4.566 |
| $0.98 | 9 | $0.94 | 4.606 |
| $0.99 | 9 | $0.94 | 4.667 |
Results
We can minimize the worst case for the number of coins needed to give change if we replaced the $0.50 coin with one of twenty-three possible alternatives. These are: $0.12, $0.14, $0.16, $0.17, $0.18, $0.19, $0.21, $0.22, $0.23, $0.24, $0.26, $0.27, $0.28, $0.29, $0.31, $0.32, $0.33, $0.36, $0.37, $0.38, $0.41, $0.42, $0.43
Any one of the solutions above minimizes the maximum regret. If, instead of a $0.50, we had any of the above coins, the worst case for the number of coins we'd have to give out is six (which is two less than with the half dollar). How do we decide which one to go with?
|
Well, this depends on your definition of 'best'. We could select the $0.32 coin which has the lowest value for the average change size (assuming we had to give out change equally likely for all values $0.01-$0.99, then a coin with a $0.32 would minimize number of coins we'd have to count out in total). Alternatively, if we wanted to minimize the number of times we had to give out six coins in change, then we should select the $0.22 coin. For this coin, only when we had to give out change for $0.19 would we need six coins; all other change values require five or less coins. (For $0.32, we need to had our six coins for both $0.19 change and $0.24) |
 |
(It's refreshing to see that if N=$0.01, $0.05, $0.10 or $0.25, the results are the same as before. If we add a coin the same as we already have, it makes no difference!)
More
 Image: Jude Freeman Image: Jude Freeman |
In American football (NFL rules), any score is possible except one*. The only way to score one point is by a single point conversion after a six point touchdown. As two points are awarded for a safety and three points for a field goal, all other scores apart from one are possible. If you liked this article, you might like to learn how much you are worth in quarters, which explains some of the history about the relationship between the dime, quarter and half-dollar. Or this article about the two egg drop problem (a classic interview problem). |
*Except if a game is forfeit, in which case the score is recorded as 1-0.
You can find a complete list of all the articles here. Click here to receive email alerts on new articles.
Click here to receive email alerts on new articles.