
	Advertisement

Fan Direction

We’re going through a bit of an early heatwave here in Seattle. Temperatures are unseasonably warm. Many stores have sold out of portable air-conditioning units, but you can still buy fans.

People are opening their windows and placing their fans next to them.

Here’s a question for you though: Is it better to blow or suck? Should you turn the fan on so that it blows fresh air into the room, or turn the fan around so that it sucks the hot air out of the room? (or does it not make any difference?) Ask three people, and you might get three different answers. I’ll give you my answer in a moment.

Is it better to suck or blow?

(If you are a PC builder you might have been involved in similar debates. Is it better to suck the hot-air out of your PC case, or blow cold air in? Maybe this article will answer that debate too!)

Hot room

Image: kevin_wen

For my analysis, I’m going to assume the temperature outside your house is lower than the temperature inside (otherwise why would you be opening the window!?!)

Solar radiation from the sun heats the inside of the house and this heat is trapped in the house because the inside of the house radiates heat back at longer wavelengths, and this can’t pass back through the windows or insulated walls. In addition, electrical equipment inside your home (lights, computers, TVs, fridges, stereos …), all turn a good chunk of their input power into heat energy!

I am going to neglect, for this analysis, the heat generated by the fan motor itself. (The fan motor generates heat as a by-product of turning the blades; if the fan is blowing out, it fairly efficiently carries this heat away). The difference caused by this is second order compared to the other effects (probably), so which side of the fan the motor is situated is not going to impact the answer I give.

Actuator Disk Theory

To answer this question, we're going to have to learn how fans work. This requires some theory and a little math (but not too much).

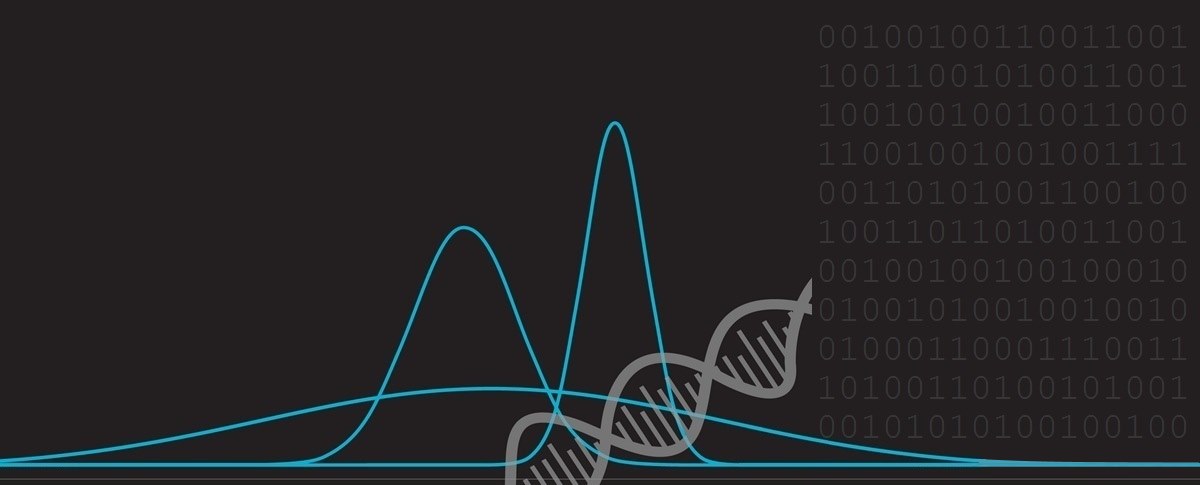
Air is a fluid. A fan is used to accelerate this fluid from stagnant (non-moving) to the velocity it has downstream of the fan. A simple model for how this process behaves is called Actuator Disk Theory. (Also sometimes called Momentum Theory, or a hybrid of the two names).

The simplified diagram to the right shows what we're going to model. On the left (upstream), air is pulled into the fan, accelerating through it to the right (downstream). The fan is shown as a blue disk.

There are some assumptions we are going to make to get our basic approximation of what happens:

As we're not dealing with massive speeds (low Mach number), we can assume the fluid is not compressible and inviscid (having zero, or negligable friction). We're assuming there are no frictional drag losses.
The fan is approximated as an 'actuator disk'. It is deemed to be created from an infinite number of blades, each with an infinite aspect ratio. It simply produces thrust by virtue of a pressure difference from one side of the disk to the other.
There is no rotation of the fluid in the slipstream; it simply passes through the disk.
There is a divide in the fluid between molecules that pass through the fan disk, and those that do not. This is called the stream tube or slipstream. Flow outside the stream tube is at stagnant pressure (no work imposed on it).
We're going to look at this in 1D with rotational symmetry..

Now the math …

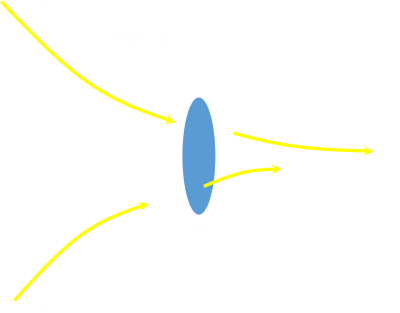
OK, now let's label a few more things:

The stagnation pressure in the room is P_∞
The area of the fan (actuator disk) is A_F
The velocity of the air flowing through the fan is V_F
The Area of the upstream stream tube and the corresponding velocity are A_U and V_U
The Area of the downstream stream tube and the corresponding velocity are A_D and V_D
The fan generates a Thrurst of T
The pressure on the upstream side of the disk is P_FU
The pressure on the downstream side of the disk is P_FD

The first equation we can write is a simple one of conservation of mass. Since nothing passes in/out the stream tube boundary, and the air is of constant density, then:

We can immediately learn something from this. Because the velocity air upstream of the fan is zero (V_U=0), then this makes the area of the stream tube (A_U) infinitely large.

A fan 'pulls' air into into itself from an, essentially, infinitely large pool of air at zero speed, slowly accelerating it towards the disk.

Next we can use conservation of energy to write an equation (using Bernoulli) for the upstream part of the stream tube. A fluid can trade its energy between dynamic pressure (kinetic energy) and static pressure (potential energy). We can't perform this calculation across the disk because pressure is being added, but here is the equation for the upstream part. On the left is the total energy far upstream, and on the right is the total energy just infront of the disk:

Because we know the upstream velocity is zero, this simplifies to give us equation (1)

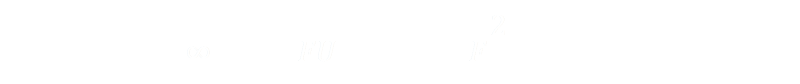
Similarly we can create an equation for the conservation of energy for the downstream part of the tube to give us equation (2):

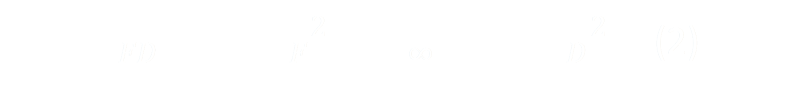
We can subtract equation (1) from equation (2)

With simplification we can see that the result is agnostic to the ambient pressure.

The fan generates thrust (even though it is mounted to a stand so does not move), and this is equal to the pressure difference over the disk, multiplied by the area of the disk:

Another way to describe the thrust is the momentum change of the air; this is the mass flow of the air, multiplied by the velocity (when it has stopped accelerating, downstream). The mass flow is the density of the air, multiplied by the velocity of the air and area of the stream tube. All these can be combines and substituted for the thrust and simplified down:

This gives us a very important result: The velocity of the air downstream of the fan is twice that of the air passing through the fan. The air accelerates up to the fan, and continues to accelerate downstream of the fan until it reaches the same pressure of the air outside the stream tube.

The velocity of the air downstream of the fan is twice that of the air passing through the fan

From conversation of mass we also know that the area of the stream tube downstream of the fan is half of the area of the fan.

The stream tube downstream is half the area of the fan

What the fan has done is funnelled a massive volume of upstream air into a very tight tunnel of fast moving air. What are the consequences of this? Well, if you happen to have the luxury of standing directly downstream of the fan in the sweetspot you'll get a wonderful benefit from this fast tube of air, but if you are outside this, other than secondary mixing, you'll not get benefit.

Conclusion

If you want to cool one particular place, by all means, point the fan directly at it, and that is the best to get maximum effect from the high velocity air. If there is only you in the room, you can selfishly point the fan at yourself for maximum local effect. If, however, you want to cool down the entire room, it's probably best to turn the fan around and get it to suck the heat from the room (hopefully the air removed will be replaced by cooler fresher air that creeps if from all around). We know from the equations above that the fan will 'draw' air from the entire room and push it out of the window as a narrow tube of warm air.

Whilst you might not know it, you've encountered this phenomenon before. Every time you blow out a candle!

Have you ever tried to suck out a candle? (I bet you pursed your lips and tried sucking just now, as you are reading this!) It's practically impossible. No matter how hard you suck, the stream tube is pulling in air from very large region so that the velocity of over the candle flame is very small; you're never going to do it.

Conversely, even small pressure difference at your pursed lips as you blow produces a very tight tube of air of focussed air at high velocity.

It's practically impossible to suck out a candle!

The same logic applies to cooling your PC. If there is one particular component that it is essential to keep cool (such as the critical component of a high-end graphics card), then yes, point the fan at that one location for maximum local effect. If, however, your goal is to keep the whole PC cool, then turn around the fan and have it evacuate the case. A suction fan will draw air from all over the case (helping keep the entire space cool as fresh air seeps in from the ventilation holes to replace the air removed). It might also help pull dust of out the entire case (which is a good thing too).

Helicopters

Another common application of actuator disk theory is the basic simulation of powered lift vehicles (like helicopters). This is how I first learned about the theory. If you imagine turning a fan so that it points downwards, the Thrust generated can be used to generate lift.

It's a pretty simple concept. If a helicopter can displace (push) the same mass of air through the disk as it weighs, then it will hover. If it can push more, it will climb upwards, if less, then if will sink down. (This is a great simplification, as things get more complicated when not in hover. If you are moving, then the upstream velocity is no longer zero. Similarly, if you are descending there are (potentially very dangerous) issues such as you can descend into your own slipstream, or wake).

Hover Efficiency

There are a whole spectrum of ways to push the desired mass of air through the disk. You can take a very large area disk and give the air just a small amount of velocity, or you can take a small area disk and give it a very large velocity. Both of these can give the same mass flow rate, both of these can cause the vehicle to hover, but the devil is in the efficiency.

To accelerate air, you need to give it energy. The higher the change in speed needed, the higher the energy needed. Once the air has done it's work for you, any excess energy it has is wasted (it's not doing anything useful for you!). It's much more efficient to take a large volume of air and give it small change in speed, than a small volume and give it a high change of speed.

This is why helicopters are very efficient at hovering. They have large rotor areas. The air passing through a helicopter rotor disk is given a (relatively) small acceleration and so, when it passes through and it's work done, it's not travelling particularly fast and does not have too much excess energy. The larger the rotor area the better (for hover efficiency). The larger the area, the small the velocity needed. Of course then you hit other limits such as structural weight, mechanical limitations and other compromises with regards to forward speed, manoeuvrability and practicaility. Like all engineering challenges, it's a trade off.

Compare a helicopter to a Harrier Jump jet. A jump jet has to force a air out of its four nozzles (which have small areas), at very high velocities. A jump jet is incredibly, incredibly, inefficient at hovering. Once the air has passed through the nozzles it has immense kinetic energy which is essentially wasted and does nothing useful.

Image: Geoff Lang

Of course, a Jump Jet can (or could; sadly not many are flying anymore) do many things that a helicopter can't. It also makes a glorious noise …

Back to your room

Efficiency applies to your room fan too. Given a choice, you should select the largest diameter fan available for your needs. Not only will it be the most efficient, it will probably be the quietest solution too.

You can find a complete list of all the articles here. Click here to receive email alerts on new articles.