The Best Full House
What is the best possible Full House you can be dealt in Poker?

We’ll assume that we are playing straight poker. You have five cards, and there are no community cards, and no other cards are visible. If you could push a magic button and be dealt any five cards, which five cards would you select to give you the best chance of winning?
If you are not familiar with poker ranks and their probabilities, you can read this article on how to calculate poker ranks (or this article which rates the chances of hands occurring if there are wild cards).
For this exercise, there are no wild cards, and it’s a standard 52 card deck.
What five cards would you pick to make Full House with the best chance or winning the hand?
Advertisement:
The Wrong Answer
There’s a good chance you probably selected Aces over Kings; something similar to that shown below.

This is the wrong answer!
It's a natural mistake to make, after all Aces over Kings is the highest ranking Full House. However it is not the hand that maximizes your chance of winning. The actual answer is Aces over Nines (or Eights, Sevens, or Sixes). Can you see why?
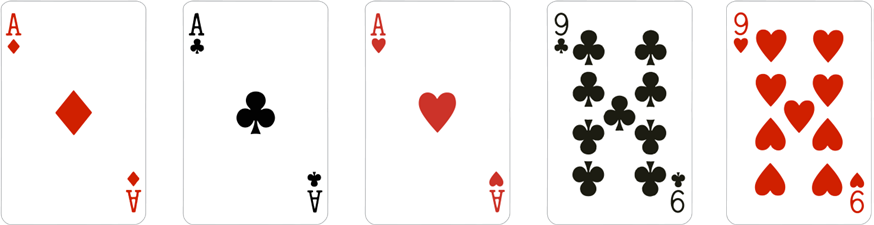
Why AAA99?
A Full House is a strong hand, and beats the all hands that are below it. However, it is vulnerable to the hands that rank above it. These hands are: Four of Kind, and Straight Flush (A Royal Flush is just a special name for the top ranking Straight Flush).
Full Houses are ordinally ranked in strength by the triplet first, followed by the pair. If you are holding three Aces, as there are only four Aces in a deck (and no community cards), then nobody else can have three Aces. A Full House with three Aces is guaranteed to be the strongest Full House, agnostic as to what the pair is. The pair doesn't need to be Kings to make the 'best' Full House; they can be any pair. A Full House with Aces over anything beats any other Full House, and all hands below.
What we need to consider is how to optimally select the pair so as to minimize the number of hands above us that could beat us.
There is nothing we can do about the Four of a Kind hands. It does not matter what we select as the pair as this does not change the odds. Our hand will use two distinct card values leaving eleven ranks to make Four of a Kind. Whatever we select for the pair does not change this.
However, the value we select for the pair can affect the number of possible Straight Flushes it is possible for an opponent to have. To make a Straight Flush, all the cards need to be of the same suit. Here, without loss of generality, are the Hearts:

I've included the Ace at both ends because, in Poker, an Ace can be either High or Low. To make a Straight Flush, there need to be five contiguous cards, as shown below:
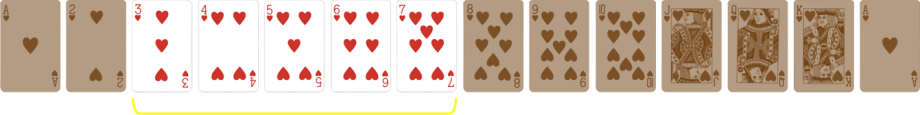
With both the Ace and King missing from a suit, the cards left mean that a possible of seven Straight Flushes can be made, from 2-3-4-5-6 through to 8-9-T-J-Q.
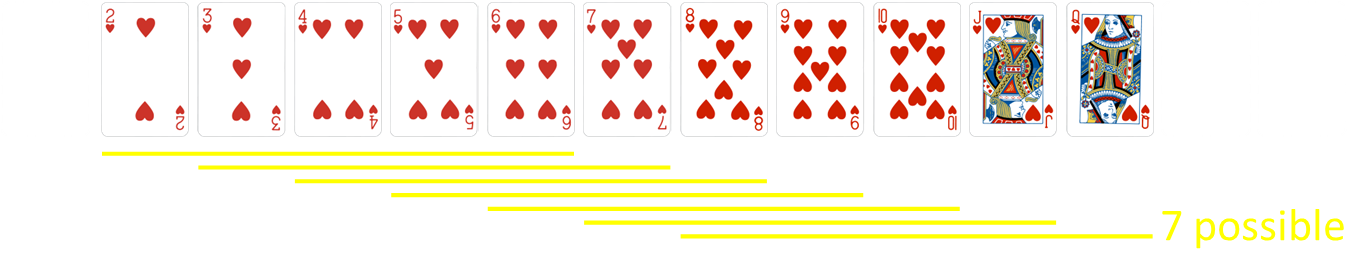
However, if we remove the Ace and the Nine, the cards left can only make three possible Straight Flushes: 2-3-4-5-6, 3-4-5-6-7, and 4-5-6-7-8 (We could deny any Straight Flush if we remove the Five and Ten, but we need the Ace to be one of the cards removed because we need this to ensure the nut Full House).
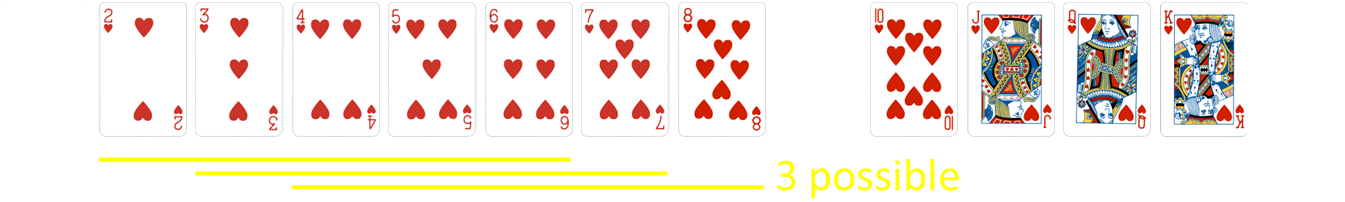
A9 is not the only solution. This also works with A8, A7, A6. These cards also deny the same number of Straight Flushes.
Selecting one of these four middle cards to be the pair reduces the number of hands that can beat us.
Suits can make a difference
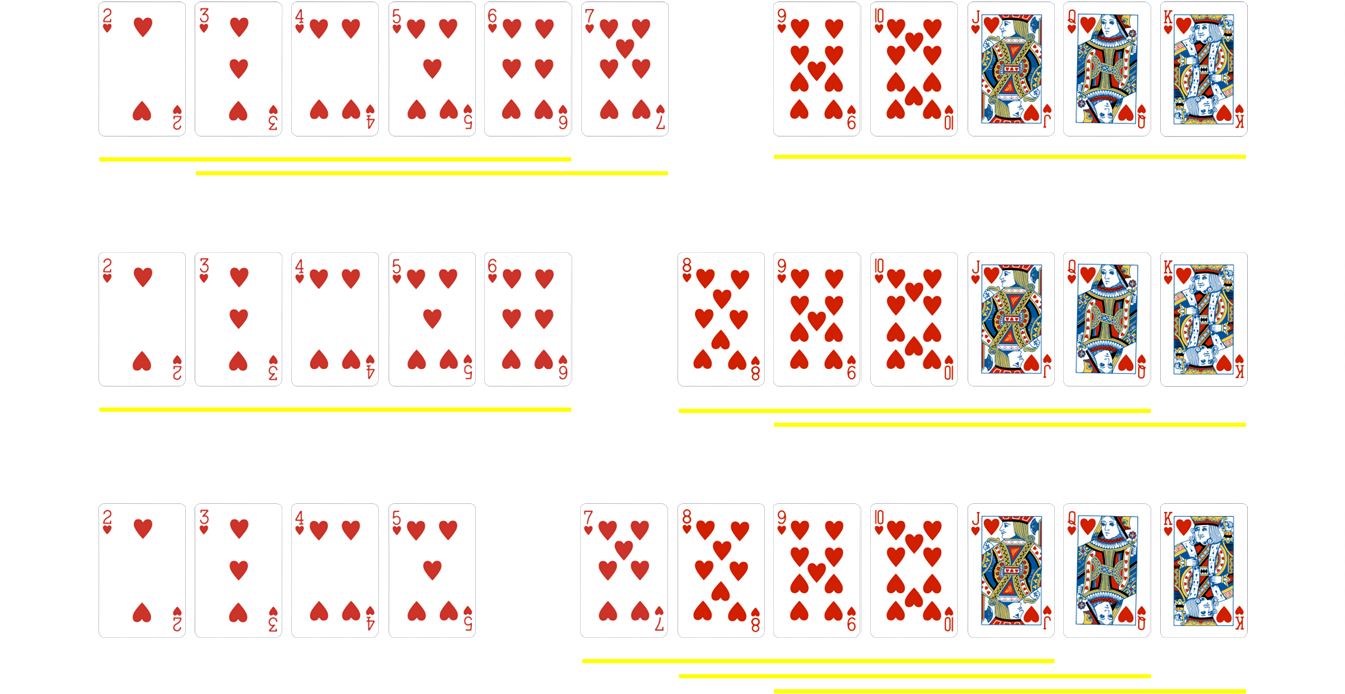
Just how much of an advantages does the correct selection give us? It's small, but measurable. Also, the selection of suits makes a slight difference. Of the five cards, the three Aces, by definition, will be from distinct suits. However, for the pair, there are two cases: The first is where the suits of both of the pair match suits with the Aces, and in the second case, one of the pair can be from a suit not yet seen.
Without loss of generality, here are the two cases and the subscript refers to a distinct suit. Let's consider the Ace/King solution first.
Case #1: A1 A2 A3 | K1 K2
Case #2: A1 A2 A3 | K1 K4
Case #1
- For suit 1, both the Ace and King are taken from the suit. This means that a possible seven Straight Flushes can be made from that suit. (7)
- Similaly, for suit 2, both the Ace and King are again taken. There can only be seven Straight Flushes possible from that suit. (7)
- For suit 3, only the Ace is missing. With just the Ace missing there are eight possible Straight Flushes: 2-3-4-5-6 through 9-T-J-Q-K. (8)
- Finally, for suit 4, as there are no missing cards, all ten natural Straight Flushes are possible: A-2-3-4-5 through T-J-Q-K-A. (10)
This results in a total of 7+7+8+10 = 32 possible Straight Flushes from this configuration.
Case #2
- For suit 1, as before, both the Ace and King are taken from the suit. This means that a possible seven Straight Flushes can be made. (7)
- For suit 2, Only the Ace is taken. There are eight Straight Flushes possible from that suit. (8)
- The same for suit 3. Eight possible Straight Flushes. (8)
- Finally, for suit 4, just the King is missing, allowing eight possible Straight Flushes: A-2-3-4-5 through 8-9-T-J-Q. (8)
This results in a total of 7+8+8+8 = 31 possible Straight Flushes from this configuration.
In the Case #1, not all suits are represented, and this results in a slightly poorer hand.
A9
Now let's consider the A9 cases:
Case #1: A1 A2 A3 | 91 92
Case #2: A1 A2 A3 | 91 94
Case #1
- For suit 1, both the Ace and Nine are taken from the suit. This means that a possible three Straight Flushes can be made from that suit. (3)
- Similaly, for suit 2, there can only be three Straight Flushes possible. (3)
- For suit 3, only the Ace is missing. With just the Ace missing there are eight possible Straight Flshes: 2-3-4-5-6 through 9-T-J-Q-K. (8)
- Finally, for suit 4, as there are no missing cards, all ten natural Straight Flushes are possible A-2-3-4-5 through T-J-Q-K-A. (10)
This results in a total of 3+3+8+10 = 24 possible Straight Flushes from this configuration.
Case #2
- For suit 1, both the Ace and Nine are taken from the suit. This means that a possible three Straight Flushes can be made from that suit. (3)
- For suit 2, Only the Ace is taken. There are eight Straight Flushes possible from that suit. (8)
- The same for suit 3. Eight possible Straight Flushes. (8)
- Finally, for suit 4, just the Nine is missing, allowing five possible Straight Flushes: A-2-3-4-5 through 4-5-6-7-8, and T-J-Q-K-A. (5)
This results in a total of 3+8+8+5 = 24 possible Straight Flushes.
The Results
All other things being equal, selecting AAA99 results in eight* fewer hands that can beat our Full House compared to AAAKK. It also does not matter what the suits are for the Nines.
Surprisingly, Aces over Nines is a better hand to wish for than Aces over Kings!
*Or nine if all suits are not represented.

Happy New Year
Happy New Year to all my readers!
