Rocket Science
I like rockets. I studied them at University. Last week I posted a little about the stability of firework rockets, and some counterintuitive conclusions from the science. This week I’m going to talk about bigger rockets, and how we use them to leave our planet.

Gravity
To escape from the Earth, a rocket must overcome the force of Gravity. Gravity is a universal force, and acts between bodies possessing mass. Gravitation forces are always in attraction (that we know of so far), and act along a line joining the centers of mass of the bodies. For this simplified view we’re going to consider the planet Earth as one mass, and give it the symbol M, and the rocket as the other, which we’ll give the symbol m.

The gravitational force exerted by an object is proportional to its mass. Mass is just another word for matter or ‘stuff’. The more massive an object, the greater the force. The gravitational force falls off with the square of the distance away (sometimes called an inverse square relationship). These effects are observations, not derivations, and they just happen, so we call them “laws”. Combining them we get the following well know formula for the Force of attraction law between the two masses:
Here, G is a constant (called, not surprisingly, the Gravitational Constant, with a value of approximately 6.674 × 10-11 Newtons kg-2 m2), and r is the distance between the two centers of mass.
If we set the value of m=1 (a unit mass), and r=RE (the radius of the Earth) we get the equation for the Force acting on a unit mass on the surface. We call this value g. Plugging in appropriate values for the mass of the Earth (in Kg), and the Radius (in meters):
You are probably familiar with this value from school.
You can see from the above equation that the value of g changes with RE, and I’ve written about this effect before. There are variations in the force of gravity depending on your distance from the center of the planet. When you are at the top of a mountain, for instance, you weigh less than at the bottom. Also, different places on Earth have different values for g based on, not just their altitude, but because the Earth is an oblate spheroid and not a perfect ball.
Variation of g
The value of g decreases inversely as the square of the distance from the center of the Earth. We can calculate the value of g at any distance r from the center of the Earth:

Dividing this equation by the equation for the value on the surface we get:
This shows the value of the gravitational force for any distance r greater than RE (inside the Earth, things are different, but a good rocket is not going in that direction!).
"Weightlessness"
A rocket leaving Earth has to overcome the force of gravity pulling it back. Note: The force of gravity never becomes zero; it just that it gets smaller and smaller. Please ignore the totally bogus ‘science’ sites that tell you that there is no gravity in space! In low Earth orbit gravity is still around 90% of what it is on the surface. Astronauts feel 'weightless' because they are constantly ‘falling’ around our planet (as is everything else around them), just like being in a free falling elevator that never reaches the bottom.

However far a rocket travels from the Earth, the Earth’s gravity is always trying to make it return. We can never escape the Earth’s gravity (“slip the surly bonds of Earth”), but we can escape the Earth.
We can never escape the Earth’s gravity, but we can escape the Earth!

Even though, if we plotted a graph of g against distance r, it would go on forever (and get closer and closer to the axis, but never touch it), the area under that curve is fixed and finite. This area represents Energy. If we give the rocket enough energy it can forever leave the Earth. It will still be pulled back and experience the incessant tug, but will have enough energy to overcome this, and never have to return.
Give me Energy
The purpose of the rocket is to give its payload sufficient energy to escape. Strictly speaking we should really be talking about ‘escape energy’ for planet Earth, but as we will see below, we make some approximations and simplifications and convert this to an ‘escape velocity’
Work (energy) is the product of force and displacement. Energy is required to move the rocket against the force of gravity. Using calculus and simple integration, we can sum up the work required to move a unit mass from the a distance r from the center of the Earth to infinity. This is the ‘area under the curve’.

We derived earlier the equation for how gr changes with r:
If it is to arrive at infinity with zero velocity, all kinetic energy from the launch should be converted to potential energy. If the rocket has mass m, then:
An object, leaving from r from the surface of the Earth, with speed Ve, will have just enough energy to never come back. To leave from the surface of the Earth r=RE, then this simplifies to:
Note: This equation is independent of mass. The escape velocity for a Humming Bird is the same as that of a Battleship.
Plugging in values:
That’s 11.2 km/second, or 25,000 mph, or about 7 mile per second!, or about Mach 33 (33× the Speed of Sound at sea level).
You need to be going pretty fast to leave the Earth!
This calculation is based on the simplification that rocket expends its energy in a short distance, and that there is no air resistance, making it a projectile. You can leave the Earth at a much slower speed, if you can afford it! There is no, theoretical, reason why you can’t crawl out of the planet at a snail’s pace. It’s just a practical reason: You’d need to burn an infeasibly massive amount of fuel constantly overcoming gravity low down that you’d not be able to carry enough fuel to get you very far off the ground before it’s all used up. (On the other hand, if you could build a fuel-less ‘glider’ to which you could ‘beam’ energy to from the ground, and somehow reflect it, you could take as much time as you needed, provided you had the energy budget. Or Maybe you could use this beamed energy to give a very small mass of fuel on the craft an insane amount of velocity to react against. We’re now moving into Science Fiction, and outside the scope of this article about rockets). The problem is not really about getting high enough to get into space, it’s about going fast enough to stay there! (once your engine stops).
You can see the vast amount of energy needed to get a rocket into space. Energy is conserved, so if a payload wants to come back, this energy has to be dissipated. The gravitational potential energy of the craft, as it returns, is converted into kinetic energy; It speeds up! Then it encounters the atmosphere and all this speed with the friction drag from the air makes it get very hot. I’m sure you’ve seen pictures of re-entry craft glowing white hot as they barrel into the Earth. Again, think conservation of energy; think of all that fuel that was burned lofting the craft into space, now imagine that being converted into heat as it comes back. Again, because of weight constraints, you can’t afford to bring fuel up with you in order to give yourself a powered descent; you pretty much have to rely on drag alone.

Orbit or Escape
If a rocket achieves a speed of the escape velocity (or greater), it will leave the Earth, and never come back. There’s another interesting speed, and this is speed needed for stable circular orbit. This is less than the escape velocity. If you give your craft more speed than the circular orbit velocity, but less than the escape velocity, it will move in an elliptical orbit. The higher the excess speed, the more eccentric the orbit will be.
For a satellite in circular orbit, the force exerted by gravity must be equal to the centripetal force required to pull it around.

If you look closely, this is 1/√2 of the escape velocity. At any location in the Earth’s gravitational field, the escape velocity from that location is √2 times the circular orbit velocity.
If you study conic sections, an object travelling at Vc will travel on a circular path. As we mentioned above, if this speed is increased, the orbit can be turned elliptical; it is still a closed path. The more the speed increases, the further the other focus of the ellipse moves away (the first focus remains in the center of the Earth), making the orbit more and more eccentric. When the velocity reaches Ve, the other focus is at infinity, and the curve becomes parabolic, and open ended. A further increase in energy and velocity turns that path into a hyperbola (the craft will still have excess energy, even at an infinite distance from the Earth).
Advertisement:
The Ideal Rocket Equation
All of this preamble leads us to the ideal (simplified) rocket equation, named after the pioneering Russian engineer Konstantin Tsiolkovsky; considered one of the founding fathers of rocket science, engineering, and astronautics. (Tsiolkovsky published his work, independently, in 1903, but it was also documented as early as 1813, by the British scientist William Moore).
A rocket is a momentum machine. Rocket engines spit out exhaust with high velocity from one end and, because of the principle of conservation of momentum, the rocket reacts in the opposite direction. However, things are not quite so simple because the fuel is consumed as the engine fires. This consumption changes the mass of the rocket over time.
Again we’ll use m to describe the mass of the rocket at any time t.
We’ll use c to describe the velocity of the rocket exhaust. We’ll assume this is fixed and constant through the burn of the rocket.
We’ll use v to describe the velocity of the rocket at time t.
From Newton’s Second Law, Force = mass × acceleration, so we can describe the relationship between the Thrust and change of velocity of the rocket as:
This is also equal to the rate change of change of momentum of the exhaust gases, which is: (Note the negative sign because this is in the opposite direction, and c is constant)
Combining:
When t=0, the rocket is about to launch, and its mass is m0. This mass is comprised of three basic components.
- Mass of fuel: mf
- Mass of payload: mp
- Mass of structure: ms
The structure is everything else that is not fuel or payload: The skin, engines, pumps, computers, safety equipment, structural members, wiring …
m0 = mf + mp + ms
At burnout m1, just the payload and structure remain. All the fuel has been burned accelearing the rest of the mass.
m1 = mp + ms
If this burn takes places over t seconds, the velocity will have increased from 0 to Vb, and we can integrate:
The numerator in the log term is simply the entire mass of the fully laden rocket. The denominator is the mass of the rocket less all the fuel burned. You will often see the burnout term described as delta-v (depictng the change in velocity the craft eperienced). As we will see leter, delta-v's are a measure of the energy required to perform a maneouvre. In space, energy is everything, and delta-v's can be stacked. It's all a conservstion of energy game. Energy gets traded beetween kinetic energy and gravitational potential eneergy.-
We can describe the mass ratio R as a measure of the efficiency of a rocket. It describes how much more massive the vehicle is with propellant than without. It is the ratio of the rocket's wet mass (vehicle plus contents plus propellant) to its dry mass (vehicle plus contents).
 Image: NASA
Image: NASA
Mass we no longer need
Looking at the ideal rocket equation, it's key that the mass ratio is critically important. The lighter we can make the structure, the more payload we can carry for the same mass of fuel. If we can reduce the structural mass as we go along, then we get the exponential bonus of less mass, and less fuel needed to accelerate this mass, and less fuel to accelerate this less fuel, etc …
This is the idea behind staging.
The concept of staging is a little like Matryoshka Dolls, but stacked ontop of each other (or sometimes in parallel). The lowest stage(s) have big engines, and big fuel tanks. They are used to launch the vehicle, with everything above it on its shoulders, up to an altitude where they have expended all their fuel. They then get jettesoned, and all that structural mass leaves the rocket (In old days, they just fell to the ground wasted, but the clever folks at SpaceX have found a, reliable, way to leave just a little fuel in them so that they can fall back to Earth, be steered, make a couple of powered burns, and then land again so that they can be resused. This is so cool).
Staging lowers the structural mass left, increasing R for what is left, improving the efficiency. A higher percentage of the remaining mass is the payload, not useless structure.
The way SpaceX return of their lower stages is so cool; you have to watch a video of this happening if you have not seen it. Here is a video of a brace of lower stages returning back to Earth; at the same time! – If you can't watch this and get a huge grin on your face, you are not an engineer.
It's not all roses. Multistage rockets require duplication of many components. There are also considerably more failure points and things to go wrong. However, at the current time, multi-stage is the only way to go (if you want any useful payload).
Staging really helps, and is the only effective way to get mass off the Earth, despite these additional complexities. There are currently no single stage to orbit (SSTO) launch vehicles*.
(*Because we are talking about leaving Earth. On the Moon, gravity is about 1/6th of that here on Earth. The Lunar Module, when it departed to rendezvous back with the Command Module used an SSTO design).
There are other benefits to staging too. The engines (and more importantly the nozzles) of the higher stages can be optimized to the atomspheric (or semi-atomspheric) conditions that they are operating under. It's a topic for a later article, but the design (shape) of the nozzle is critical to the efficiency of the engine and how it attempts to equalize the pressure so that as much of the momentum of the gas leaving the engine points directly rearwards). Lower stage engines are also larger, and if they are gimballed to steer and stablize the craft, these mechanisms, and their supporting structures, are larger too …
Futility of Single Stage Rockets
Going back to the ideal rocket equation we see that the ultimate delta-V (change in speed of the rocket) is dependant on the rocket exhaust gas speed. We already calculated the delta-V we need to leave Earth (it's fixed irrespective of mass of the vehicle - you can't negotiate with physics).
The next constant in the ideal rocket equation is c, the exhaust gas velocity. Again, we can't negotiate with physics (or chemistry and thermodynamics); the rocket muzzle velocity has a fixed maximum value. Even using the best kind of chemical rocket with the highest exhaust speed (Hydrogen as fuel), the combination of these two constants dicates the mass ratio R. If we don't build a rocket with better than this mass ratio, it will not get to where we need to send it!
You can't negotiate with physics!
Limiting Mass Ratio
A Hydrogen/Oxygen rocket has an exhaust gas velocity of around 4,500 m/s (the highest of all the chemical engines). Inverting the ideal rocket equation we can calculate the minimum mass ratio R=eVb/c. The delta-v required to reach escape velocity is 11,816 m/s, and so this gives an ideal mass ratio of close to 14 (and forget about it if you are using Kersone/Oxygen engines with exhaust speeds of 3,000 m/s which equates to an R value of over 50). (Now, if you wanted to risk putting a nuclear reactor in your rocket, and dispensing with the chemical rocket, then these engines been tested to produce exhaust velocities in excess of 8,000 m/s. But now you have other challenges and safety concerns …)
Here is a list of R for a few common types of vehicle for comparison to single stage (I'm also showning the mass of the propellant as the percentage of the total mass of a fully loaded vehicle):
| Vehicle | R | Mass Percentage Propellant | |
|---|---|---|---|
| Ship | 1.03 | 3% | |
| Truck | 1.03 | 3% | |
| Car | 1.04 | 4% | |
| Train | 1.08 | 7% | |
| Military Jet | 1.43 | 30% | |
| Passenger Jet | 1.67 | 40% | |
| Theoretical Single Stage Rocket (Circular Orbit – Hydrogen Oxygen Engine) | 6.67 | 85% | |
| Theoretical Single Stage Rocket (Earth Escape Velocity – Hydrogen Oxygen Engine) | 14.29 | 93% |
Here is a plot of the achievable delta-v for various values of R. There are lines for various exhaust gas velocities from 1,000 through to 5,000 m/s. Note the log scale for R.
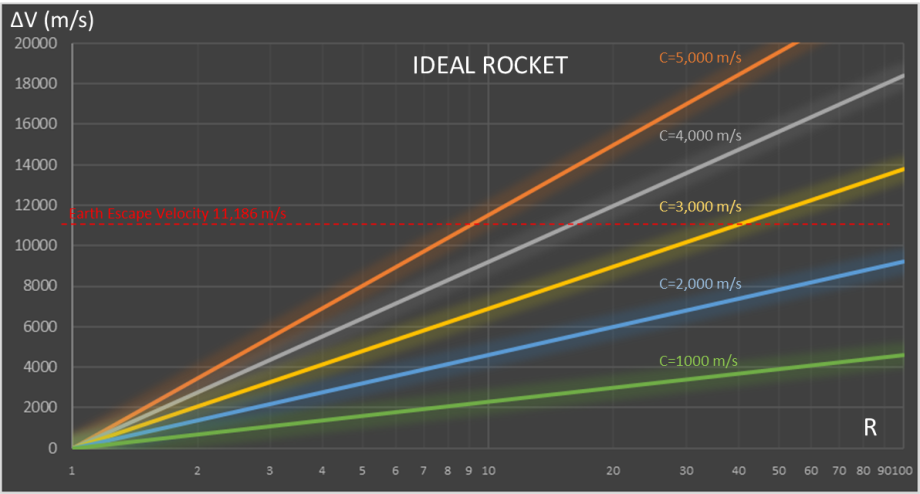
Sadly, the physics gets worse for our poor terrestrial launched rocket …
But we're not ideal
The above calculations are based on the “ideal” rocket equation. This assumes there are no other forces acting on the rocket (as if the rocket were operating deep in interstellar space). To accelerate to this speed takes time. The fuel has to not only provide the energy to accelerate the craft to the desired speed, but the reaction motor also has to provide the energy to overcome gravity until it gets there. We have seen how gravity deviates with altitude so this calculation is more complex and we’d need to know the time of the burn and the acceleration profile. Also, the rocket will not be moving just ‘vertically’ (perpendicular to the surface) all the time, but, if aiming for an orbit, will gradually be turning to convert the speed into the tangential velocity needed for orbit. Gravity force still points ‘down’ but the thrust, and thus acceleration of the craft, will be at an ever changing angle. This increases R
The ideal rocket equation ignored many things to give a simplified approximation. A rocket leaving the surface of the Earth has to battle other forces. In deep space, all we are concerned about is Thrust, and the Ideal Rocket Equation holds. Leaving the Earth requires the rocket to overcome its weight. We describe this battle as the Thrust/Weight ratio. If this ratio is less than 1.0 the rocket will just make an expensive noise on the launch pad. To move, the ratio needs to be greater than one. The higher the ratio, the faster the rocket will accelerate. A high value of this ratio will help accelerate the rocket quicker to the desired delta-v to perform the manoeuvre required. It's a balancing act; too low a T/W ratio, and the rocket will slowly claw its way into space, spending a long time in gravity and burn more fuel. Too high a T/W and the rocket may get too fast too soon, increasing the aerodynamic pressure on the craft and causes dangerous loads. Also too high an acceleration may damage fragile crew or payloads. As the rocket burns, we know it losses mass, so acceleration increases. Ideally the rocket engines should be modulated to adjust their thrust to what is needed in that part of the flight regime. Theorotically, the most efficient speed to be at, at any time, is the current terminal velocity of the craft in the current aerodynamic conditions, but usually this speed is exceeded to get the payload ascent faster so that it will be travelling faster higher up.
The ideal rocket equation is modified by the additional forces acting on the craft. As it works its way up through the atmosphere, it is constantly battling gravity. The longer it takes, the more fuel is burned. The integral term depends on the duration of the burn, and this is based on the T/W ratio and the throttling and the trajectory. Remember, however, that we learned that, close to the Earth, gravity does not vary much? (In low Earth orbit, the strength of gravity is still 90% of that at sea level, so as a first approximation, we can just assume an average value for g. It's the time that the rocket is battling against this gravitational force that is dominant. Getting up there quickly minimizes the gravitational loss (infact, this is what the Ideal Rocket Equaation calculates; a purely projectile approach in which all the delta-v is given as an impulse to launch the vehicle at the desired speed).
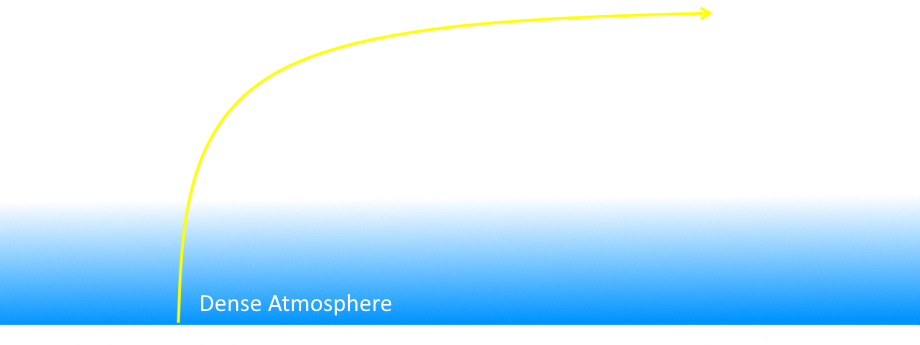
Strictly speaking for the gravity loss we should also be using a resolved component as the rocket going into orbit will slowly transition from vertical (radial acceleration), to the tangential acceleration so that it can achieve the desired velocity for orbit.
There's another term in this eauation and this is the velocity loss due to air drag. For large rockets, which accelerate slowly in the lower dense atmosphere, and actually spend a small amount of time there (the density of air rapidly drops), the total drag velocity is usually less than 1% of the delta-v, and can be effectively ignored. (However, if you are firing a small rocket with a high initial acceleration, such a small sounding rocket, this term becomes more significant).
Because the atomsphere gets so thin so quickly, aerodynamic drag (and lift), reduces rapidly*. To help reduce strucutral weight the fairing of the rocket is jetesoned into the climb as soon as possible. Because there's neglibale dynamic pressure when the cowl is jetesoned, the high drag coefficient of an unshielded payload compared to the low drag coefficient of a smoothly faired nose cone is unimportant. The loss of mass, however, is signficant.
*There is a 70% atmospheric pressure top at the peak of Mt Everest (9km). At 80% drop for a cruising commercial jet liner. By 15km, the drop is 90%, and by 30km it's a 99% loss. The Earth's atomsphere is pretty thin in context.
All these terms reduce the delta-v from the ideal equation. This is why staging is so essential to get any kind of payload into space.
A modern rocket, such as the Falcon Heavy, with its staging, can get a payload of about 1% to escape velocity.
Efficiency
Gravity is a cruel mistress. Just to leave the Eearth and get into Earth orbit takes an immmese amount of energy. Paraphrasing the astronaut Don Pettit, if you can get into Earth orbit, you are pretty much half-way to anywhere else in the Solar System. It takes the same amount of energy to get from the ground to 400km above the Earth as it does to get from this 400km location to Mars!
If you can get into orbit, you are halfway to anywhere else in the Solar System!
Neil Armstrong's first step onto the Moon might was certainly a giant leap for mankind, but it was an even bigger leap to get his Eagle and fellow crew members into orbit!
 Image: NASA
Image: NASA
It’s staggering just how much fuel is needed to get a small payload to leave the Earth. Staggering. Remember the dry mass of the rocket (stuff that is not fuel), is both the payload and the structure.
Just putting this into context, a 95% fuel precentage is approximately the same ratio as soda is to a can of soda. A full can of Coke weighs approximately 384g, and any empty can weighs just 15g. Just 4% of the weight of a full can of Coke is the can! Imagine this scaled up to rocket size. A can is a pretty passive skin holding your beverage. In a rocket, this percentage needs to provide the structure to hold cryogenic fuel in tanks (against it trying to boil off), the skin of the rocket, the engines, computers, pumps and structure to keep the rocket together (oh, and also the payload!)

Think of a rocket like a giant can of Coke. The beverage inside is the propellant. They empty can represents the rocket and payload.
Think of a rocket like a giant can of Coke.
How lucky are we?
Returning to the escape velocity equation:
We can see that the escape velocity is entirely dependant on the mass of the planet and it's radius. Whilst it's pretty brutal from Earth, if we lived on a slightly denser planet, or a slightly larger planet with the same density (mass goes up with the cube of the radius), then we quickly reach a point that it's practially impossible to leave using a rocket. There are planets out there, if they are inhabited, that it will be impossible for the inhabitants to leave by rocket (unless they decide to convert the entire mass of their planet into propellent). Brutal is better than impossible; Earthlings should consider themselves lucky! Here's a fun little paper if you want to learn more: SUPER-EARTHS IN NEED FOR EXTREMLY BIG ROCKETS.
Many extra-terrestrial aliens may never be able to leave their planets!
Staging Math
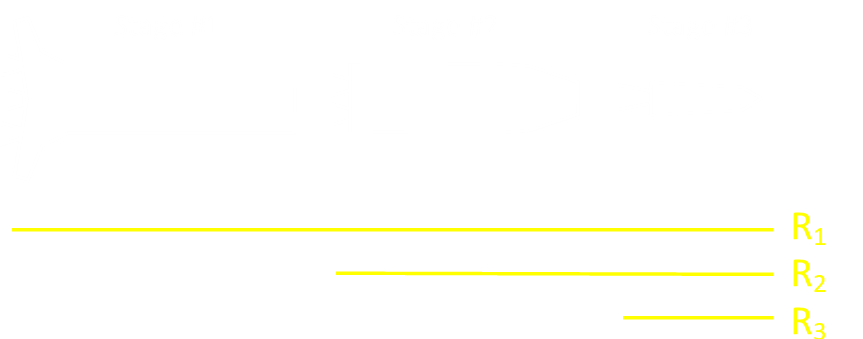
Staging is the way to dump mass that is no longer need. As an example above is a three stage rocket.
In this example, the rockets are fired in series. It’s like the first stage carries a smaller rocket up until it runs out of fuel, then a second smaller rocket takes off from this end point carrying an even smaller rocket on its shoulders. Finally the third stage fires. The delta-v’s are added.
The final stage reaches the velocity of the sum of the velocities of each separate stage giving an effective mass ratio R=R1R2R3. Staging therefore effectively increases R, and hence Vb.

Stages can use different propellenats and engines. A vertical climb of 100 seconds causes a delta-v of around 1,000m/s from gravity loss, so the lower stages want a larger T/W ratio to minimize ascent time. This is why you often see launch vehicles with strap on solid-boosters on their lower stages; these have higher impulse than liquid fueled rockets.
 Image: NASA
Image: NASA
Getting up or staying up
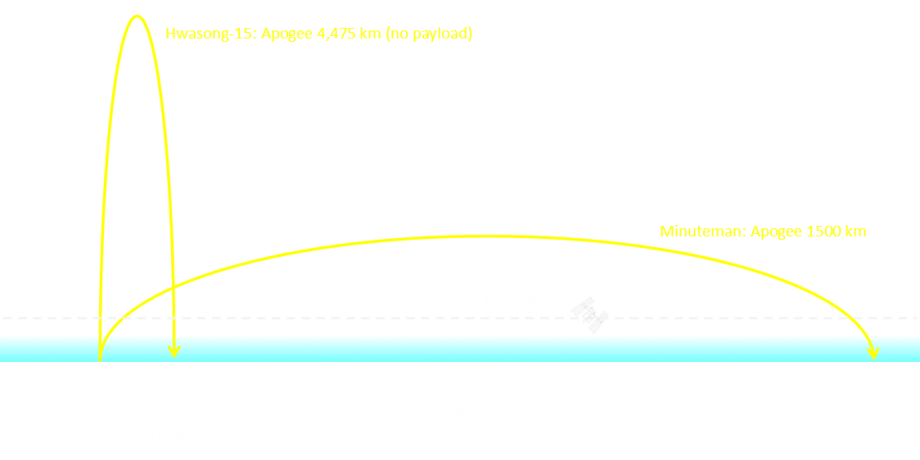
The energy required to stay in orbit is much higher than simply getting into space. This can be clearly illustrated by looking at Intercontinental Ballistic Misssiles. Something like the US Minuteman has a range of around 10,000km (probably), and to get there, it's stages boost the craft to an apogee of around 1,500km (probably). This is much higher than the ISS, which is hurtling through space a just 408km altitude.
The Minuteman is also carrying a reasonably massive nuclear payload (probably), and if the target is is closer, it can loft it's apogee higher.
I've also plotted the estimated flight path of the North Korean Hwasong-15 ICBM they are developing. I'm showing this for a couple of reasons; the first is that their test was (probably) not carrying any payload at all. If you don't need any payload you can dedicate all non-structure mass to extra fuel. If their craft was carrying a heavy payload, as we've learned from the rocket equation, it's performance would be greatly reduced. Second you can see that, if you don't care about giving your craft tangential velocity, you an use all the energy to make it go up, and not worry about sideways. You can see it boosted itself to an order of magnitude altitude than the ISS; but what goes up, must come down. The third is that they did not attempt to go for range (just 1000km along), so most of there energy went into going up, not along, which is another reason for the impressive looking altitude.
Tolerances
Because things are so close to the limit, there is not room for much safety. Quoting again from astronaut Don Pettit, from his lecture on the Tyranny of the Rocket Equation:
"Another less recognized side effect of the rocket equation is the sensitivity of completing the rocket burn to obtaining your goal. To illustrate this, I will use some numbers from my Shuttle flight, STS 126 in November 2008. Our target velocity at main engine cut off was 7824 m/s (25819 ft/s). If our engines shut down at 7806 m/s (25760 ft/s), only 18 m/s (59 ft/s) shy of the target value, we would make an orbit but not our designated target orbit. We would not be able to rendezvous with space station and would lose our mission objective. Like being two pennies short of a ten dollar purchase, this is only 0.2% less than the price of admission into space. In this case, we do have some options. We could burn our orbital maneuvering propellant and make up this difference. If we were 3% shy of our target, 7596 (25067 ft/s) we would not have sufficient orbital maneuvering propellant and we would not make any orbit. We would be forced into a trans-Atlantic abort, falling back to Earth and landing in Spain. This final 3% of our required velocity comes during the last 8 seconds of our burn. For astronauts and bull riders, 8 seconds is a long time."
It's a similar issue with engineering. To get the maximum payload possible, every possible structural gram is removed. This cuts down safety margins. Stresses on a rocket can have safety margins down to scarily low percentages. If your car is rated to travel at 70 mph, you'd expect it to safely be able to drive at 80 mph without failing catastrophically. A similar percentage delta to a rocket as it passes through the atmosphere could rip it apart. We really are at the limit of many technologies with rockets.
Rocket Science (and engineering) is hard!
