Second Place?
We’re all pretty familiar with the single elimination tournament system. It’s the standard bracketing system used by many sports. A plurality of teams enter an event, pair off and, at each game, one of the teams loses and goes home; the other advances. But, how fair is it?
Each round eliminates half of the remaining teams, and (depending on the number of entrants) reduces down to quarter-final brackets, semi-final brackets and ultimately the final game. The victor of the final match is declared the winner of the tournament (quite rightly so; they have won every single game they played).
Second place is given the loser of the final game, but how fair is that? What is the probability that the second best team also makes it to the final? It could have been that the second best team met the ultimate winners very early on in the competition and their untimely departure ensured they were not able to progress to the final.

What is the probability that the second best team makes it to the final?
Advertisement:
Seeding
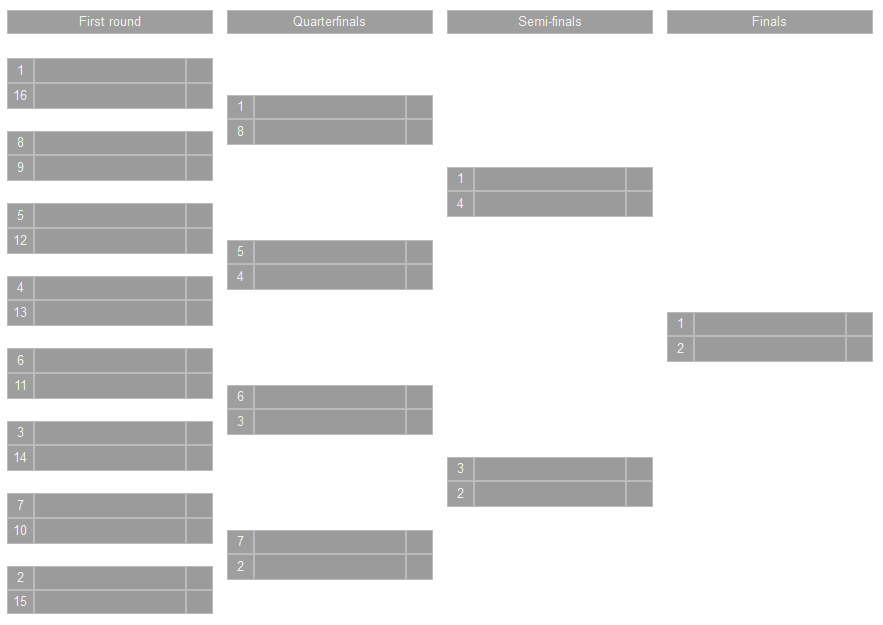
If you have knowledge of the rankings of the teams, and the teams are seeded going into an event, they can be striped to try and maximize the chance that the second best team graduates to the final. This is achieved by pairing off the best teams in descending order against the worst teams in ascending order (The highest seeded team plays against the lowest ranked team. The second highest ranked team plays against the second to lowest ranked team …)
These pairings are initially planted in the bracket in a position, so that, at each stage if the highest ranked team advances, they are still paired off in this way (and the lowest ranked half of the seedings is eliminated at each round). An easy way to see this is to look at the rounds in reverse order, and see that, in a recursive way, the rounds are nested, like Matryoshka Dolls.
Here is an example seeded bracket for sixteen teams.
Elimination
In a single elimination tournament it is sudden death. If you lose any game, you go home. As two teams play each game, and one team leaves, on each round the field is halved. This means that the number of teams that start the competition needs to be an exact power of two. If your event has a natural power of two entrants, perfect. If not, the next highest power of two needs to be selected and these additional slots are padded with “bys” (the other team automatically progressing), which are simply phantom teams with a ranking below the lowest ranked team in the event.
Because one team is eliminated on every game, if a tournament starts with n players (e.g. 32 players), then n-1 games need to be played (one team leaves on each game, until one remains).
Second Place
Returning to the original question, let’s assume there is no prior knowledge of the teams, so no seeding.
If we assume that in any round that higher skilled team always wins its game, then we can see that the only way for the second placed team to advance to the final is if it is in the other ‘half’ of the bracket from the highest skilled team.
If teams #1 and #2 ever meet before the final, then the second skilled team will be eliminated at that point. The probability that the second ranked team will make it to the final is the probability that it was placed in the opposite half of the bracket from the top team.
Formally
Because a perfect bracket needs to have 2x teams, then we can say that out of these teams, then 2x/2 teams are in each half of the bracket. This is 2x − 1.
The number of entrants to the event is 2x, so there are 2x−1 starting slots that are not the ultimate winner. So the probability of the second skilled team being in the other half of the bracket to the winning teams is 2x−1/2x − 1
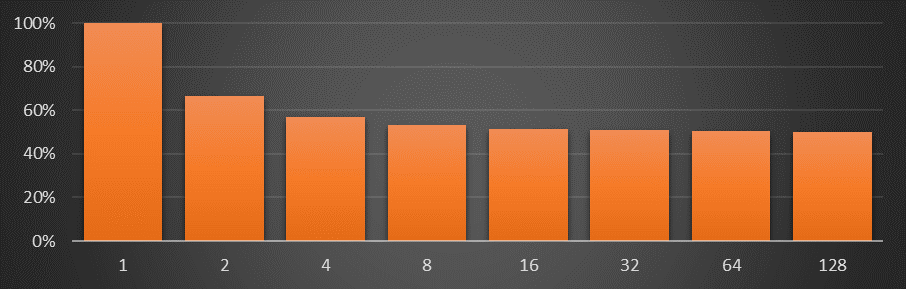
Here is some data in tabular form, and the percentage chance.
| Teams | Starting Positions (2x−1) | Half Bracket Size (2x − 1) | Chance of #2 in final | |
|---|---|---|---|---|
| 1 | 0 | 0 | — | |
| 2 | 1 | 1 | 100.000% | |
| 4 | 3 | 2 | 66.667% | |
| 8 | 7 | 4 | 57.143% | |
| 16 | 15 | 8 | 53.333% | |
| 32 | 31 | 16 | 51.613% | |
| 64 | 63 | 32 | 50.794% | |
| 128 | 127 | 64 | 50.394% | |
| 256 | 255 | 128 | 50.196% |
You can see that, as the competition gets larger, the probability that the second skilled team makes it to the final asymptotes to 50%
In a large event, there’s only approximately 50% chance that the true second best team makes it to the final.
All we can say for certain is that the team that was ‘runner-up’, and placed second, was the winner of its half of the bracket. In the most extreme case, its ordinal position is one less than half the teams (Imagine an evil bracket in which all the good teams are in one half, and all the bad teams in the other. The 'best of the worst' will bubble up to the final).
There are similar issues with 3rd and 4th placing as many large competitions have an additional playoff round for the respective losers of the semi-finals to determine their rankings. It’s very possible for the team to place 3rd being ‘better’ than the team placing 2nd.
It’s not all negative comments for single elimination tournaments. On the positive side, they are very efficient, paring down the field by half on every round.
Fixing the problem
There are a couple of ways to address some of the limitations of single elimination events. If the teams are seeded then it’s possible to ‘re-seed’ after each round so that the highest surviving team gets to play the lowest surviving team in the next round, and so on. But this is a hack based on knowing the seeding, and shows that seedings are not perfect anyway as there is variability in games (after all, if seedings were perfect and matches pre-ordained, how was it possible for an under-dog team to advance and justify the need for a re-balancing of the bracket?)
A better way is the double elimination tournament.
Double Elimination
In a double elimination event, a team does not leave the competition until it has lost two matches. This has many advantages. The first is that it does not penalize a team for a freak tragic loss; it is still possible to win the tournament, even if you have one loss.
It’s also more fun for the teams (who might have travelled a long distance), as they are guaranteed at least two games (remember, otherwise, half the teams will have to go home after just one game!).
A double elimination also ensures that the top two teams will meet in the final irrespective of where they started. (Even if the top two teams clash early, the winning team will stay in the top bracket, and the second place team will be relegated to the lower bracket where it will dominate, and the two teams will meet again in the final).
The double elimination system works by using two virtual brackets (sometimes called winners and losers brackets, or upper and lower brackets). The winner bracket behaves just like the single elimination bracket above, but this time, the loser does not go home, but instead descends to the loser bracket. In the lower bracket, a win propagates you forward, but a loss from this bracket send you home.
A downside of a double elimination bracket is that there are more games needed. If n teams enter, than the number of games required increases to 2n−2 (or possibly 2n−1, if the winning team never loses a game). Because of the variable number of games related to the finals, it makes scheduling harder. An additional bonus is the a 3rd place playoff round is not needed as those results come for free already.
Below is an example bracket for 8 teams. All teams start in the upper bracket. The winners advance as normal. Numbers on the graph represent the matches played, and the letter/number combinations represent where the loser of a game moves to.
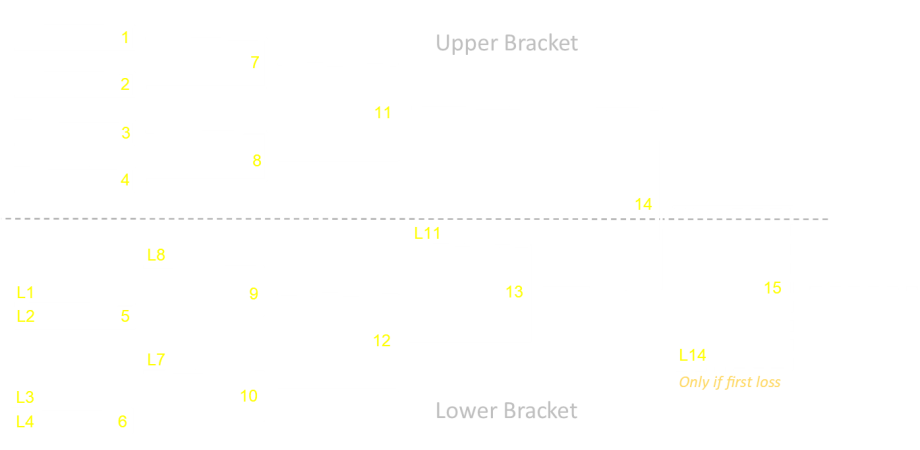
If the ultimate winner of the game 14 has not lost, the tournament is over after 14 games. If, however, for the loser of game 14 is their first loss they get to play again (after all, this is double elimination, and a team can only be eliminated after two losses). It is possible to win the tournament even after one loss (whenever that loss is)!
Much fairer!
