Two sixes in a row

As my good friend, Bryan, would say, I’m on a roll with writing about dice probability.

Here is today’s puzzle: You go to a discount store and buy a large quantity of six-sided dice. You then put a number of them in a bucket, give the bucket a good shake, and tip them out onto the table. How many dice do there need to have been in the bucket so that there is a 50% chance of getting at least one six? How many should you put in the bucket to have 95% chance (confidence) that you’ll get at least one six? How many for a 99% chance?

How many dice do you need to roll to get at least one six with 95% confidence?

Bernoulli Trials

Statisticians describe experiments like this as a collection of Bernoulli trials. Bernoulli trials are events that only have two possible outcomes: Success/Fail (Flipping a coin, rolling a six or not, are you pregnant?, do you have a disease? Was your card the three of clubs? …) You may also see these referred to as Dichotomous responses.

There is a 1/6 chance that any die will roll a six. Conversely, there is a 5/6 chance it will not. All the dice rolls are independent. If we wanted to calculate the chance of getting a specific number of sixes we could work out the number of combinations of Successes and Failures. If we did this, we’d end up with something called a Binomial Distribution of outcomes (which is a topic for a whole different article).

In our experiment, though, things are a lot simpler as we are looking for one specific case: We’re looking for at least one six. The complement of this is no sixes. If we calculate the chance of rolling no sixes, we can subtract this from 1 to get the chance of rolling at least one six.

The chance for any single die rolling a non-six is 5/6. For n independent trials, the chance of every dice being a non-six is (5/6)ⁿ. Therefore the chance of getting at least one six is 1-(5/6)ⁿ.

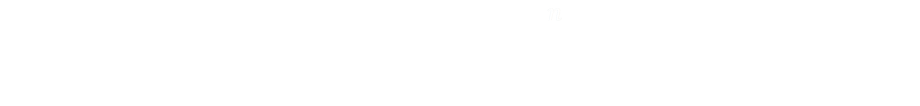
This gives the probability (confidence) for a specified number of dice. What we want is the other way around. A quick bit of algebra converts it to the formula we need.

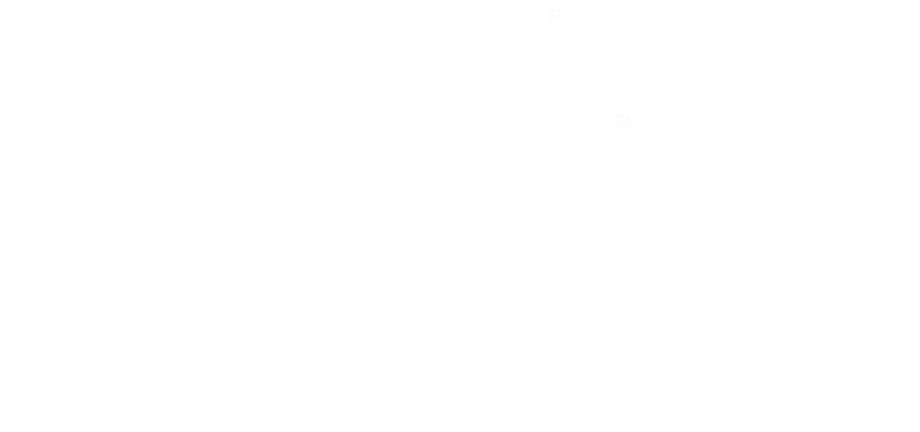
Plugging in a value of Pr=0.5, give the results n≈3.8, since dice are quantized, this means we’d need to roll four dice. For 95% confidence, Pr=0.95, n≈16.43, so we’d need at least 17 dice.

How about other numbers?

99.9% (“three nines”) n≈37.89
99.999% (“five nines”) n≈63.15
99.9999999999999% (“fifteen nines”) n≈189.44
99.99999999999999999999999% (“twenty five nines”) n≈315.73

If you have a bucket of a couple of hundred dice, you can be pretty certain that you’re going to get at least one six!

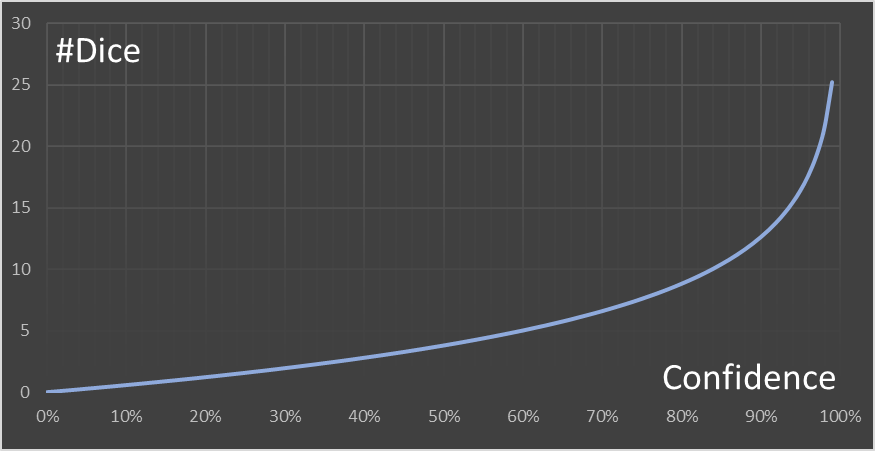
Here’s a graph showing how the confidence changes with the number of dice rolled.

Social Distancing

The global pandemic is on everyone’s mind at the moment. There are many opinions about just how viral this is, and the mortality rate, and plenty of people are attempting to model it and map out the exponential growth. I’m not an expert in any of these things, and the last thing the world needs is another unqualified opinion. We should let expert epidemiologists guide us with their (peer reviewed) analyses.

That said, we can apply what we have learned from the dice, and Bernoulli trials, to understand the importance of social distancing. We’re not going to model the growth of the virus, just highlight what your behavior does to your chances of getting infected.

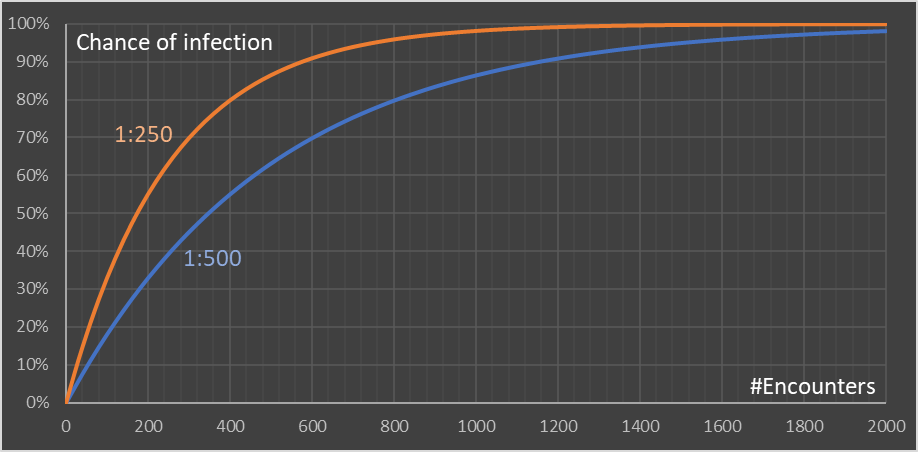
This is a great simplification; see the caveats above, but let’s imagine that each person you encounter in a day has a chance of being infected. Let’s say that the level is currently 1:500 (one in every five hundred people has the virus), and if you meet anyone that is infected you get infected too. You can’t be double infected, so once you are infected that’s it (this is like rolling ‘at least’ one six).

If you are currently virus free, if you don’t interact with anyone, you remain virus free.
If you meet one external person, there is a 499/500 you will remain virus free. The chances of you being infected are 1-(499/500) = 0.2%
If you meet two external people, your chance increases to 1-(499/500)² = 0.3996%
If you meet ten external people, your chance increases to 1-(499/500)¹⁰ = 1.98%
If you travel on a bus and interact with fifty external people, your chance is 1-(499/500)⁵⁰ = 9.53%. You have to dodge fifty, independent, bullets.
To get to 50:50 chance on infection, it’s an encounter with 346 people.
Just because the infection rate is 1 in 500, it does not mean that if you encounter 500 people you are certain to get it. If you encounter 500 people, your chances are 63.25%

Here is the data graphed out. I’ve also included the curve for if the infection level is 1:250

Stay safe.